How to prove that the sides of a quadrilateral are equal. Parallelogram
Quadrilateral ABCD is a figure that consists of four points A, B, C, D, three each, not lying on the same straight line, and four segments AB, BC, CD and AD connecting these points.
The pictures show quadrilaterals.
Points A, B, C and D are called vertices of a quadrilateral, and segments AB, BC, CD and AD - parties. Vertices A and C, B and D are called opposite vertices. Sides AB and CD, BC and AD are called opposing parties.
There are quadrilaterals convex(left in the picture) and non-convex(in the picture - right).
Each diagonal convex quadrilateral divides it into two triangles(diagonal AC divides ABCD into two triangle ABC and ACD; diagonal BD - on BCD and BAD). U non-convex quadrilateral only one of the diagonals divides it into two triangles(diagonal AC divides ABCD into two triangles ABC and ACD; diagonal BD does not).

Let's consider main types of quadrilaterals, their properties, area formulas:
Parallelogram
Parallelogram is called a quadrilateral whose opposite sides pairwise parallel.
Properties:
Signs of a parallelogram:
1. If two sides of a quadrilateral are equal and parallel, then this quadrilateral is a parallelogram.
2. If in a quadrilateral the opposite sides are equal in pairs, then this quadrilateral is a parallelogram.
3. If in a quadrilateral the diagonals intersect and are divided in half by the point of intersection, then this quadrilateral is a parallelogram.
Area of a parallelogram:

Trapezoid
Trapeze A quadrilateral is called a quadrilateral in which two sides are parallel and the other two sides are not parallel.
Reasons are called parallel sides, and the other two sides are sides.

Middle line A trapezoid is a segment connecting the midpoints of its sides.
THEOREM.
middle line the trapezoid is parallel to the bases and equal to their half-sum.

Trapezoid area:

Rhombus
Diamond is called a parallelogram in which all sides are equal.
Properties:
Rhombus area:

Rectangle
Rectangle is called a parallelogram in which all angles are equal.
Properties:
Rectangle sign:
If the diagonals of a parallelogram are equal, then this parallelogram is a rectangle.
Rectangle area:

Square
Square is called a rectangle whose sides are all equal.
Properties:
A square has all the properties of a rectangle and a rhombus (a rectangle is a parallelogram, therefore a square is a parallelogram with all sides equal, i.e. a rhombus).
Square area:




















 Back forward
Back forward
Attention! Slide previews are for informational purposes only and may not represent all the features of the presentation. If you are interested this work, please download the full version.
The purpose of the lesson: consider the characteristics of a parallelogram and consolidate the acquired knowledge in the process of solving problems.
Tasks:
- educational: developing the ability to apply parallelogram features to solve problems;
- developing: development logical thinking, attention, skills independent work, self-esteem skills;
- educational: nurturing interest in the subject, the ability to work in a team, a culture of communication.
Lesson type: learning new material, primary consolidation.
Equipment: interactive board, projector, task cards, presentation.
During the classes
1. Organizational moment.
U: Good afternoon, guys! Today in class we will again talk about parallelograms. We have to complete many tasks, prove theorems and learn how to apply them when solving problems. The motto of our lesson will be the words of Le Carbusier: “Everything around is geometry.”
2. Updating students' knowledge.
Theoretical survey
Give some students individual assignments on cards on the topic properties of a parallelogram(everyone chooses tasks independently on the presentation slide via a hyperlink, pointing the mouse pointer at the figure, but not at the number), listen individually to each respondent.
With the rest - prove additional properties of a parallelogram. (First discuss the proof orally, then check it with the interactive whiteboard).
1°. The bisector of the angle of a parallelogram cuts off an isosceles triangle from it.
2°. The bisectors of adjacent angles of a parallelogram are perpendicular, and the bisectors opposite angles are parallel or lie on the same straight line.
After preparation, listen to evidence of additional properties of a parallelogram.
ABCD -parallelogram,
AE is the bisector of angle BAD.
Prove: ABE is isosceles.
Proof:
Since ABCD is a parallelogram, therefore BC || AD, then angle EAD = angle BEA lying crosswise with parallel lines BC and AD and secant AE. AE is the bisector of angle BAD, which means angle BAE = angle EAD, therefore angle BAE = angle BEA.
In ABE, angle BAE = angle BEA, which means ABE is isosceles with base AE.
Suggestive questions:
Formulate the sign of an isosceles triangle.
Which angles in BAE can be equal? Why?
ABCD -parallelogram,
BE is the bisector of angle CBA,
AE is the bisector of angle BAD.
Suggestive questions:
When will lines AE and CK be parallel?
Are angles BEA and<3? Почему?
In what case will AE and CK coincide?
Preparing to study new material
Frontal work with the class (orally).
- What do the words “properties” and “character” mean? Give examples.
- What is the converse theorem?
- Is the opposite of this statement always true? Give examples.
3. Explanation of new material.
U.: Each object has its own properties and characteristics. Please tell me how properties differ from signs.
Let's try to understand this issue using a simple example. The given object is autumn. Name its properties: Its characteristics:
- What statements are the property and attribute of an object in relation to each other? (answer: inverse)
- What properties have we already studied in the geometry course? State them. (name a few)
Is it possible to construct a true converse statement for any property? (different answers).
Let's check this on the following properties:
Conclude: Is it possible to construct a true converse statement for any property? (no, not for anyone)
Now, let's return to our quadrilateral, remember its properties and formulate their converse statements, i.e.:.. (answer - characteristics of a parallelogram). So, the topic of today's lesson is: “Signs of a parallelogram.”
So, name the properties of a parallelogram.
Formulate statements that are inverse to the properties. (students formulate signs, the teacher corrects them and formulates them again)
Let us prove these signs. The first sign is in detail, the second is brief, the third is on your own at home.
4. Consolidation of the studied material.
Work in workbooks: solve problem No. 11 on the interactive whiteboard, call a less prepared student to the board.
Solution to problem No. 379 (write the solution on the interactive whiteboard). From vertices B and D of the parallelogram ABCD, in which AB BC and A are acute, perpendiculars BC and DM are drawn to the straight line AC. Prove that the quadrilateral BMDK is a parallelogram.
Average level
Parallelogram, rectangle, rhombus, square (2019)
1. Parallelogram
Compound word "parallelogram"? And behind it lies a very simple figure.
Well, that is, we took two parallel lines:

Crossed by two more:

And inside there is a parallelogram!
What properties does a parallelogram have?
Properties of a parallelogram.
That is, what can you use if the problem is given a parallelogram?
The following theorem answers this question:
Let's draw everything in detail.
What does it mean first point of the theorem? And the fact is that if you HAVE a parallelogram, then you will certainly
The second point means that if there IS a parallelogram, then, again, certainly:
Well, and finally, the third point means that if you HAVE a parallelogram, then be sure to:
Do you see what a wealth of choice there is? What to use in the problem? Try to focus on the question of the task, or just try everything one by one - some “key” will do.
Now let’s ask ourselves another question: how can we recognize a parallelogram “by sight”? What must happen to a quadrilateral so that we have the right to give it the “title” of a parallelogram?
Several signs of a parallelogram answer this question.
Signs of a parallelogram.
Attention! Begin.
Parallelogram.
Please note: if you found at least one sign in your problem, then you definitely have a parallelogram, and you can use all the properties of a parallelogram.
2. Rectangle
I think that it will not be news to you at all that
First question: is a rectangle a parallelogram?
Of course it is! After all, he has - remember, our sign 3?
And from here, of course, it follows that in a rectangle, like in any parallelogram, the diagonals are divided in half by the point of intersection.
But the rectangle also has one distinctive property.
Rectangle property
Why is this property distinctive? Because no other parallelogram has equal diagonals. Let's formulate it more clearly.
Please note: in order to become a rectangle, a quadrilateral must first become a parallelogram, and then demonstrate the equality of the diagonals.
3. Diamond
And again the question: is a rhombus a parallelogram or not?
With full right - a parallelogram, because it has and (remember our feature 2).
And again, since a rhombus is a parallelogram, then it must have all the properties of a parallelogram. This means that in a rhombus, opposite angles are equal, opposite sides are parallel, and the diagonals bisect at the point of intersection.
Properties of a rhombus
Look at the picture:

As in the case of a rectangle, these properties are distinctive, that is, for each of these properties we can conclude that this is not just a parallelogram, but a rhombus.
Signs of a diamond


And again, pay attention: there must be not just a quadrilateral whose diagonals are perpendicular, but a parallelogram. Make sure:
No, of course, although its diagonals are perpendicular, and the diagonal is the bisector of the angles and. But... diagonals are not divided in half by the point of intersection, therefore - NOT a parallelogram, and therefore NOT a rhombus.
That is, a square is a rectangle and a rhombus at the same time. Let's see what happens.
Is it clear why? - rhombus is the bisector of angle A, which is equal to. This means it divides (and also) into two angles along.
Well, it's quite clear: the diagonals of a rectangle are equal; The diagonals of a rhombus are perpendicular, and in general, a parallelogram of diagonals is divided in half by the point of intersection.
AVERAGE LEVEL
Properties of quadrilaterals. Parallelogram

Properties of a parallelogram
Attention! Words " properties of a parallelogram"mean that if in your task There is parallelogram, then all of the following can be used.
Theorem on the properties of a parallelogram.
In any parallelogram:
Let's understand why this is all true, in other words WE'LL PROVE theorem.
So why is 1) true?
If it is a parallelogram, then:
- lying criss-cross
- lying like crosses.
This means (according to criterion II: and - general.)
Well, that’s it, that’s it! - proved.
But by the way! We also proved 2)!
Why? But (look at the picture), that is, precisely because.
Only 3 left).
To do this, you still have to draw a second diagonal.
And now we see that - according to the II characteristic (angles and the side “between” them).
Properties proven! Let's move on to the signs.
Signs of a parallelogram
Recall that the parallelogram sign answers the question “how do you know?” that a figure is a parallelogram.
In icons it's like this:
Why? It would be nice to understand why - that's enough. But look:
Well, we figured out why sign 1 is true.
Well, it's even easier! Let's draw a diagonal again.
Which means:
AND It's also easy. But...different!
Means, . Wow! But also - internal one-sided with a secant!
Therefore the fact that means that.
And if you look from the other side, then - internal one-sided with a secant! And therefore.
Do you see how great it is?!
And again simple:
Exactly the same, and.
Pay attention: if you found at least one sign of a parallelogram in your problem, then you have exactly parallelogram and you can use everyone properties of a parallelogram.
For complete clarity, look at the diagram:

Properties of quadrilaterals. Rectangle.
Rectangle properties:
Point 1) is quite obvious - after all, sign 3 () is simply fulfilled
And point 2) - very important. So, let's prove that
This means on two sides (and - general).
Well, since the triangles are equal, then their hypotenuses are also equal.
Proved that!
And imagine, equality of diagonals is a distinctive property of a rectangle among all parallelograms. That is, this statement is true^
Let's understand why?
This means (meaning the angles of a parallelogram). But let us remember once again that it is a parallelogram, and therefore.
Means, . Well, of course, it follows that each of them! After all, they have to give in total!
So they proved that if parallelogram suddenly (!) the diagonals turn out to be equal, then this exactly a rectangle.
But! Pay attention! This is about parallelograms! Not just anyone a quadrilateral with equal diagonals is a rectangle, and only parallelogram!
Properties of quadrilaterals. Rhombus
And again the question: is a rhombus a parallelogram or not?
With full right - a parallelogram, because it has (Remember our feature 2).
And again, since a rhombus is a parallelogram, it must have all the properties of a parallelogram. This means that in a rhombus, opposite angles are equal, opposite sides are parallel, and the diagonals bisect at the point of intersection.
But there are also special properties. Let's formulate it.
Properties of a rhombus
Why? Well, since a rhombus is a parallelogram, then its diagonals are divided in half.
Why? Yes, that's why!
In other words, the diagonals turned out to be bisectors of the corners of the rhombus.
As in the case of a rectangle, these properties are distinctive, each of them is also a sign of a rhombus.
Signs of a diamond.
Why is this? And look,
That means both These triangles are isosceles.
To be a rhombus, a quadrilateral must first “become” a parallelogram, and then exhibit feature 1 or feature 2.
Properties of quadrilaterals. Square
That is, a square is a rectangle and a rhombus at the same time. Let's see what happens.
Is it clear why? A square - a rhombus - is the bisector of an angle that is equal to. This means it divides (and also) into two angles along.
Well, it's quite clear: the diagonals of a rectangle are equal; The diagonals of a rhombus are perpendicular, and in general, a parallelogram of diagonals is divided in half by the point of intersection.
Why? Well, let's just apply the Pythagorean theorem to...
SUMMARY AND BASIC FORMULAS
Properties of a parallelogram:
- Opposite sides are equal: , .
- Opposite angles are equal: , .
- The angles on one side add up to: , .
- The diagonals are divided in half by the point of intersection: .
Rectangle properties:
- The diagonals of the rectangle are equal: .
- A rectangle is a parallelogram (for a rectangle all the properties of a parallelogram are fulfilled).
Properties of a rhombus:
- The diagonals of a rhombus are perpendicular: .
- The diagonals of a rhombus are the bisectors of its angles: ; ; ; .
- A rhombus is a parallelogram (for a rhombus all the properties of a parallelogram are fulfilled).
Properties of a square:
A square is a rhombus and a rectangle at the same time, therefore, for a square all the properties of a rectangle and a rhombus are fulfilled. And.
This is a quadrilateral whose opposite sides are parallel in pairs.
Property 1. Any diagonal of a parallelogram divides it into two equal triangles.
Proof . According to the II characteristic (crosswise angles and common side).
The theorem is proven.
Property 2. In a parallelogram, opposite sides are equal and opposite angles are equal.
Proof .
Likewise,
The theorem is proven.

Property 3. In a parallelogram, the diagonals are bisected by the point of intersection.
Proof .
The theorem is proven.

Property 4. The angle bisector of a parallelogram, crossing the opposite side, divides it into an isosceles triangle and a trapezoid. (Ch. words - vertex - two isosceles? -ka).
Proof .

The theorem is proven.
Property 5. In a parallelogram, a line segment with ends on opposite sides passing through the point of intersection of the diagonals is bisected by this point.
Proof .
The theorem is proven.

Property 6. The angle between the altitudes dropped from the vertex of an obtuse angle of a parallelogram is equal to an acute angle of a parallelogram.
Proof .

The theorem is proven.
Property 7. The sum of the angles of a parallelogram adjacent to one side is 180°.
Proof .
The theorem is proven.

Constructing the bisector of an angle. Properties of the angle bisector of a triangle.
1) Construct an arbitrary ray DE.
2) On a given ray, construct an arbitrary circle with a center at the vertex and the same
with the center at the beginning of the constructed ray.
3) F and G - points of intersection of the circle with the sides of a given angle, H - point of intersection of the circle with the constructed ray

Construct a circle with center at point H and radius equal to FG.
5) I is the point of intersection of the circles of the constructed beam.
6) Draw a straight line through the vertex and I.
IDH is the required angle.
)
Property 1. The bisector of an angle of a triangle divides the opposite side in proportion to the adjacent sides.

Proof . Let x, y be segments of side c. Let's continue the beam BC. On ray BC we plot from C a segment CK equal to AC.
In order to determine whether a given figure is a parallelogram, there are a number of signs. Let's look at the three main features of a parallelogram.
1 parallelogram sign
If two sides of a quadrilateral are equal and parallel, then this quadrilateral will be a parallelogram.
Proof:
Consider the quadrilateral ABCD. Let the sides AB and CD be parallel. And let AB=CD. Let's draw the diagonal BD in it. It will divide this quadrilateral into two equal triangles: ABD and CBD.
These triangles are equal to each other along two sides and the angle between them (BD is the common side, AB = CD by condition, angle1 = angle2 as crosswise angles with the transversal BD of parallel lines AB and CD.), and therefore angle3 = angle4.
And these angles will lie crosswise when the lines BC and AD intersect with the secant BD. It follows from this that BC and AD are parallel to each other. We have that in the quadrilateral ABCD the opposite sides are pairwise parallel, and therefore the quadrilateral ABCD is a parallelogram.
Parallelogram sign 2
If in a quadrilateral the opposite sides are equal in pairs, then this quadrilateral will be a parallelogram.
Proof:
Consider the quadrilateral ABCD. Let's draw the diagonal BD in it. It will divide this quadrilateral into two equal triangles: ABD and CBD.

These two triangles will be equal to each other on three sides (BD is the common side, AB = CD and BC = AD by condition). From this we can conclude that angle1 = angle2. It follows that AB is parallel to CD. And since AB = CD and AB is parallel to CD, then according to the first criterion of a parallelogram, the quadrilateral ABCD will be a parallelogram.
3 parallelogram sign
If the diagonals of a quadrilateral intersect and are bisected by the point of intersection, then this quadrilateral will be a parallelogram.
Consider the quadrilateral ABCD. Let us draw two diagonals AC and BD in it, which will intersect at point O and are bisected by this point.

Triangles AOB and COD will be equal to each other, according to the first sign of equality of triangles. (AO = OC, BO = OD by condition, angle AOB = angle COD as vertical angles.) Therefore, AB = CD and angle1 = angle 2. From the equality of angles 1 and 2, we have that AB is parallel to CD. Then we have that in the quadrilateral ABCD the sides AB are equal to CD and parallel, and according to the first criterion of a parallelogram, the quadrilateral ABCD will be a parallelogram.
 What is morphological analysis of a word: an example on all parts of speech
What is morphological analysis of a word: an example on all parts of speech What nouns have no gender?
What nouns have no gender?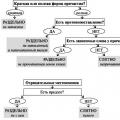 Rules for writing not with short participles
Rules for writing not with short participles