Example

Splitting a segment in half

Bisection problem. Use a compass and ruler to divide this segment AB into two equal parts. One of the solutions is shown in the figure:

• Using a compass we draw circles with centers at points A And B radius AB.
• Finding intersection points P And Q two constructed circles (arcs).
• Using a ruler, draw a segment or line passing through the points P And Q.
• Finding the desired midpoint of the segment AB- point of intersection AB And PQ.

Formal definition

In construction problems, the set of all points of the plane, the set of all straight lines of the plane and the set of all circles of the plane are considered, on which the following operations are allowed:

1. Select a point from the set of all points:
   1. arbitrary point
   2. arbitrary point on a given line
   3. arbitrary point on a given circle
   4. the point of intersection of two given lines
   5. point of intersection/tangency of a given line and a given circle
   6. points of intersection/tangency of two given circles
2. "By using rulers» select a line from the set of all lines:
   1. arbitrary straight line
   2. an arbitrary straight line passing through a given point
   3. a straight line passing through two given points
3. "By using compass» select a circle from the set of all circles:
   1. arbitrary circle
   2. an arbitrary circle with a center at a given point
   3. an arbitrary circle with a radius equal to the distance between two given points
   4. a circle with a center at a given point and with a radius equal to the distance between two given points

In the conditions of the problem, a certain set of points is specified. It is required, using a finite number of operations from among the admissible operations listed above, to construct another set of points that is in a given relationship with the original set.

The solution to the construction problem contains three essential parts:

1. Description of the method for constructing a given set.
2. Proof that the set constructed in the described way is indeed in a given relationship with the original set. Usually the proof of the construction is carried out as a regular proof of the theorem, based on axioms and other proven theorems.
3. Analysis of the described construction method for its applicability to different versions of the initial conditions, as well as for the uniqueness or non-uniqueness of the solution obtained by the described method.

Known Issues

• Apollonius' problem of constructing a circle tangent to three given circles. If none of the given circles lies inside the other, then this problem has 8 significantly different solutions.
• Brahmagupta's problem of constructing an inscribed quadrilateral using its four sides.

Construction of regular polygons

Ancient geometers knew how to construct correct n-gons for , , and .

Possible and impossible constructions

All constructions are nothing more than solutions to some equation, and the coefficients of this equation are related to the lengths of given segments. Therefore, it is convenient to talk about constructing a number - a graphical solution to an equation of a certain type. Within the framework of the above requirements, the following constructions are possible:

• Construction of solutions to linear equations.
• Constructing solutions to quadratic equations.

In other words, it is only possible to construct numbers equal to arithmetic expressions using the square root of the original numbers (lengths of segments). For example,

Variations and generalizations

• Constructions using one compass. According to the Mohr-Mascheroni theorem, with the help of one compass you can construct any figure that can be constructed with a compass and a ruler. In this case, a straight line is considered constructed if two points are specified on it.
• Constructions using one ruler. It is easy to see that with the help of one ruler only projective-invariant constructions can be carried out. In particular, it is impossible to even divide a segment into two equal parts, or find the center of a drawn circle. But if there is a pre-drawn circle on the plane with a marked center, using a ruler, you can carry out the same constructions as with compasses and a ruler (Poncelet-Steiner theorem ( English)), 1833. If there are two notches on a ruler, then constructions using it are equivalent to constructions using compasses and a ruler (Napoleon took an important step in proving this).
• Constructions using tools with limited capabilities. In problems of this kind, tools (as opposed to the classical formulation of the problem) are considered not ideal, but limited: a straight line through two points can be drawn using a ruler only if the distance between these points does not exceed a certain value; the radius of circles drawn using a compass can be limited from above, below, or both above and below.
• Constructions using flat origami. see Hujit rules

see also

• Dynamic geometry programs allow you to perform constructions using a compass and ruler on a computer.

Notes

Literature

• A. Adler Theory of geometric constructions / Translation from German by G. M. Fikhtengolts. - Third edition. - L.: Uchpedgiz, 1940. - 232 p.
• I. I. Alexandrov Collection of geometric construction problems. - Eighteenth edition. - M.: Uchpedgiz, 1950. - 176 p.
• B. I. Argunov, M. B. Balk. - Second edition. - M.: Uchpedgiz, 1957. - 268 p.
• A. M. Voronets Geometry of the compass. - M.-L.: ONTI, 1934. - 40 p. - (Popular library on mathematics under the general editorship of L. A. Lyusternik).
• V. A. Geiler Unsolvable construction problems // coolant. - 1999. - No. 12. - P. 115-118.
• V. A. Kirichenko Constructions with compass and ruler and Galois theory // Summer School “Modern Mathematics”. - Dubna, 2005.
• Yu. I. Manin Book IV. Geometry // Encyclopedia of elementary mathematics. - M.: Fizmatgiz, 1963. - 568 p.
• Y. Petersen Methods and theories for solving geometric construction problems. - M.: Printing house of E. Lissner and Y. Roman, 1892. - 114 p.
• V. V. Prasolov Three classic construction problems. Doubling a cube, trisection an angle, squaring a circle. - M.: Nauka, 1992. - 80 p. - (Popular lectures on mathematics).
• J. Steiner Geometric constructions performed using a straight line and a fixed circle. - M.: Uchpedgiz, 1939. - 80 p.
• Optional course in mathematics. 7-9 / Comp. I. L. Nikolskaya. - M.: Education, 1991. - P. 80. - 383 p. - ISBN 5-09-001287-3

Wikimedia Foundation. 2010.

See what “Construction using a compass and ruler” is in other dictionaries:

Rulers - get a working coupon for a discount on AllInstruments at Akademika or buy rulers at a profit with free delivery on sale at AllInstruments

A branch of Euclidean geometry, known since ancient times. In construction tasks, the following operations are possible: Mark an arbitrary point on the plane, a point on one of the constructed lines, or the intersection point of two constructed lines. With the help of... ... Wikipedia

Constructions using compasses and rulers are a branch of Euclidean geometry known since ancient times. In construction tasks, the following operations are possible: Mark an arbitrary point on the plane, a point on one of the constructed lines, or a point... ... Wikipedia

Noun, s., used. compare often Morphology: (no) what? construction, what? construction, (I see) what? construction, what? construction, about what? about construction; pl. What? construction, (no) what? constructions, why? constructions, (I see) what? construction, with what?... ... Dmitriev's Explanatory Dictionary

SMALL ACADEMY OF SCIENCES OF SCHOOLCHILDREN OF CRIMEA

"SEEKER"

Section "Mathematics"

GEOMETRICAL CONSTRUCTIONS USING A DOUBLE-SIDED RULER

I've done the work A

_____________

Class student

Scientific director

INTRODUCTION………………………………………………………………………………..…..3

I. GEOMETRIC CONSTRUCTIONS ON THE PLANE………………...4

I.1. General axioms of constructive geometry. Axioms of mathematical instruments………………………………………………………………………………..4

I.2. ……………………….....5

I.3. Geometric constructions with one ruler……………………………..7

I.4. Basic tasks for constructing with a double-sided ruler………………..8

I.5. Solving various construction problems …………………………………12

I.6. Constructions with a one-sided ruler………………………………….....20

I.7. Interchangeability of a double-sided ruler with a compass and a ruler....21

CONCLUSION…………………………………………………………….24

List of references……………………………..………….25

Introduction

Problems involving construction with limited means include problems involving construction using only compasses and a ruler, which are considered in the school curriculum. Is it possible to solve construction problems with only one ruler? Often you don’t have a compass at hand, but you can always find a ruler.

Problems on constructions in geometry are a fascinating section. Interest in it is due to the beauty and simplicity of the geometric content. The relevance of considering these problems increases due to the fact that they are used in practice. The ability to use one ruler to solve the problems considered in this work is of great importance in practical activities, because We are constantly faced with problems of dividing a segment in half, doubling a given segment, etc.

This paper examines the main construction problems that serve as a basis for solving more complex problems.

As experience shows, construction tasks arouse interest and contribute to the activation of mental activity. When solving them, knowledge about the properties of figures is actively used, the ability to reason is developed, and the skills of geometric constructions are improved. As a result, constructive abilities develop, which is one of the goals of studying geometry.

Hypothesis: all construction problems that can be solved using a compass and ruler can only be solved using a double-sided ruler.

Object of study: construction tasks and double-sided ruler.

Research objectives: to prove that all construction problems can be solved only with the help of a double-sided ruler.

Research objectives: to study the theoretical foundations of solving construction problems; solve basic construction problems using a double-sided ruler; give examples of more complex construction tasks; systematize theoretical and practical material.

I. GEOMETRIC CONSTRUCTIONS ON THE PLANE

I.1. General axioms of constructive geometry. Axioms of mathematical tools

For constructive geometry it is necessary to have an accurate and, for mathematical purposes, complete description of a particular tool. This description is given in the form of axioms. These axioms in abstract mathematical form express those properties of real drawing instruments that are used for geometric constructions.

The most commonly used geometric construction tools are:ruler (one-sided) , compass, two-sided ruler (with parallel edges) and some others.

A. Ruler axiom.

The ruler allows you to perform the following geometric constructions:
a) construct a segment connecting two constructed points;

b) construct a straight line passing through two constructed points;

c) construct a ray emanating from a constructed point and passing through another constructed point.

B. The compass axiom.

The compass allows you to perform the following geometric constructions:
a) construct a circle if the center of the circle and a segment equal to the radius of the circle (or its ends) have been constructed;

B. Axiom of a double-sided ruler.

The double-sided ruler allows you to:

a) carry out any of the constructions listed in axiom A;

b) in each of the half-planes defined by the constructed line, construct a line parallel to this line and passing from it at a distanceA, Where A - a segment fixed for a given ruler (width of the ruler);

c) if two points A and B are constructed, then determine whether AB will be greater than a certain fixed segmentA (ruler width), and if AB >A , then construct two pairs of parallel lines passing through points A and B respectively and spaced from one another at a distanceA .

In addition to the listed tools, you can use other tools for geometric constructions: an arbitrary angle, a square, a ruler with marks, a pair of right angles, various devices for drawing special curves, etc.

I.2. General principles for solving construction problems

Construction task consists in the fact that it is required to construct a certain figure with the specified tools if some other figure is given and certain relationships between the elements of the desired figure and the elements of this figure are indicated.

Each figure that satisfies the conditions of the problem is calleddecision this task.

Find a solution construction task means reducing it to a finite number of basic constructions, i.e., indicating a finite sequence of basic constructions, after which the desired figure will already be considered constructed by virtue of the accepted axioms of constructive geometry. The list of acceptable basic constructions, and, consequently, the progress of solving the problem, significantly depends on what specific tools are used for constructions.

Solve the construction problem - Means, find all its solutions .

The last definition requires some clarification. Figures that satisfy the conditions of the problem can differ in both shape or size, and position on the plane. Differences in position on the plane are taken into account or not taken into account depending on the formulation of the construction problem itself, on whether the condition of the problem provides or does not provide for a certain location of the desired figure relative to any given figures.

If a solution to a problem is found, then in the future it is allowed to use this solution “as a whole,” that is, without dividing it into main constructions.

There are a number of simple geometric construction problems, which are especially often included as components in solving more complex problems. We will call them elementary geometric construction problems. The list of elementary tasks is, of course, conditional. Basic tasks usually include the following:

Divide this segment in half.

Dividing a given angle in half.

Constructing on a given line a segment equal to the given one.

Constructing an angle equal to a given one.

Constructing a line passing through a given point parallel to a given line.

Constructing a line passing through a given point and perpendicular to a given line.

Division of a segment in this respect.

Constructing a triangle using three given sides.

Constructing a triangle using a side and two adjacent angles.

Constructing a triangle using two sides and the angle between them.

When solving any somewhat complex construction problem, the question arises about how to reason in order to find a way to solve the problem, to obtain all solutions to the problem, to find out the conditions for the possibility of solving the problem, etc. Therefore, when solving constructive problems, they use a solution scheme , consisting of the following four stages:

1) analysis;
2) construction;
3) proof;
4) research.

I.3. Geometric constructions with one ruler

We will consider the ruler from two points of view: as a ruler and as a double-sided ruler.

1. Double-sided ruler width A we will call a ruler with parallel edges located at a distance A from each other, making it possible to directly build:

a) an arbitrary straight line;

b) a straight line passing through two points given or obtained in the process of solving the problem;

c) parallel lines, each of which passes through one of the points, the distances between which are greaterA (in this construction, the ruler is in such a position that on each of its two parallel edges there is one of the two given points; in this case, we will talk about direct construction).

The width of the ruler in this construction is considered constant, and therefore, if in the process of solving a specific problem it becomes necessary to perform a direct construction relative to some obtained pointsA And IN , then we must prove that the lengthAB longer A .

We will consider a point to be constructed if it is one of the data or is the intersection of two constructed lines; in turn, we will consider a straight line to be constructed if it passes through the constructed or given points.

Using a double-sided ruler you can construct the following.

a) Through any two points you can draw a straight line, and only one.

b) Whatever the straight line, there are exactly two straight lines in the plane, parallel to it and separated from it by a distancea .

c) Through two points A and B at AB A it is possible to draw two pairs of parallel straight; with AB = A you can draw a pair of parallel lines, the distance between which is equalA .

If one, two, three points are given, then no new points can be constructed

(Figure 1);

if four points are given, some three of which (or all four) lie on the same line, then no other points can be constructed (Fig. 2);

If you are given four points lying at the vertices of a parallelogram, you can construct only one point - its center. (Fig.3).

Having accepted the above, let us consider separately the problems solved by a double-sided ruler.

I.4. Basic tasks for constructing with a double-sided ruler

1
. Construct the bisector of angle ABC.

Solution: (Fig. 4)

A  (IN C) And b  (AB), and  b = D .

We get B D– bisector  ABC.

Indeed, obtained by

constructing a parallelogram is

rhombus, since its heights are equal. IND –

the diagonal of a rhombus is a bisector ABC. Fig.4

2
. Double the given angle ABC

Solution : (Fig. 5) a) A  (AB),

A  (IN C)= D , through points B and D

b directly;

b) through points B andD m  b

directly,b Ç a = F .

We get Ð AB F = 2 Ð ABC .

Fig.5

3 . To a given straight line M N in this

draw a perpendicular to point A

Solution : (Fig.6)

1) (AA 1) || (BB 1) || (SS 1) –

directly (B (M N),

WITH Î (M N)); 2) through A and B

m || n - directly,

m Ç (SS 1) = D .

We get (A D )  (M N ).

Fig.6.

4
. Through a given point not lying on

given line, draw a perpendicular

To this line.

Solution: Through this point O we draw

two lines intersecting a given

straight line AB, and double the angles of the resulting

triangles adjacent to this

straight.  OA N = 2  OAV and

OB N = 2  OVA (Fig. 7).

Fig.7

5. Construct a point symmetrical to a given line relative to a given line.

Solution: see problem 4. (point O is symmetrical to pointN. Fig.7)

6. Carry out a straight line parallel to this one

P
straight M N , through point A, not

belonging to the line M N .

Solution 1: (Fig. 8)

1)(AA 1) || (BB 1) || (SS 1) || (DD 1 ) || (КК 1) -

– directly, (SA)Ç (BB 1) = C 2;

2) (With 2 K) Ç (DD 1 ) = F .

(A F ) is the desired straight line.

Fig 8

Solution 2 . In Fig. 8 1 is numbered

sequence of straight lines,

of which 1, 2 and 3 are parallel in

direct construction;

(A F) || (M N).

Fig.8 1

7
. Divide this segment AB in half.

Solution 1. (Fig. 9) (only for the case when the width of the ruler is less than the length of this segment). Draw two pairs of parallel lines directly through

the ends of this segment, and then the diagonal

the resulting rhombus. O – middle AB.

Rice. 9.

Solution 2. (Fig. 9, a)

1) a || (AB) and b || (AB) – directly;

2) (AR), (AR)Ç a = C, (AP) Ç b = D ;

3) (D IN) Ç a = M, (SV) Ç b = N ;

4) (M N ) Ç (AB) = K;

5) (D TO) Ç (A N ) = F ;

6) (B F ) Ç b = D 1, (B F ) Ç a = C 1;

7) (D IN ) Ç (A D 1 ) = X,

(AC 1) Ç (SV) = Z.

8) (X Z) Ç (AB) =O. We get AO = OB.

Fig.9,a

Solution 3 .( Rice. 9, b)

As is known , in the middle trapezoid

bases, point of intersection

diagonals and intersection point

extensions of the sides

lie on the same straight line.

1) m || (AB) – directly;

2) C Î m , D Î m , (AS) Ç (IN D ) = TO; Fig.9,b

3) (NE) Ç (A D ) = F ; 4) (K F ) Ç (AB) =O. We get AO = OB.

I.5. Solving various construction problems

In solving the following construction problems using only a double-sided ruler, the direct construction of parallel lines and the seven main problems given above are used.

1. Draw two mutually perpendicular lines through this point.

R solution: let's pass through this point

two arbitrary lines,

and then - bisectors

adjacent corners. (Fig.10)

Fig.10

2. Given a segment A D given length a.

Construct a segment whose length is equal to .

R
decision : Let's carry out m  A And h || m through

point A. f || (A D ) , k || (AD) directly.

Let's draw AB and AC, where B =f  m ,

a C = m  k . In a known way

divide AB and AC in half and

let's draw the medians of the triangle

ABC. By the property of medians

triangle, O D = – sought

segment (Fig. 11)

Rice. eleven

3. Construct a segment whose length is

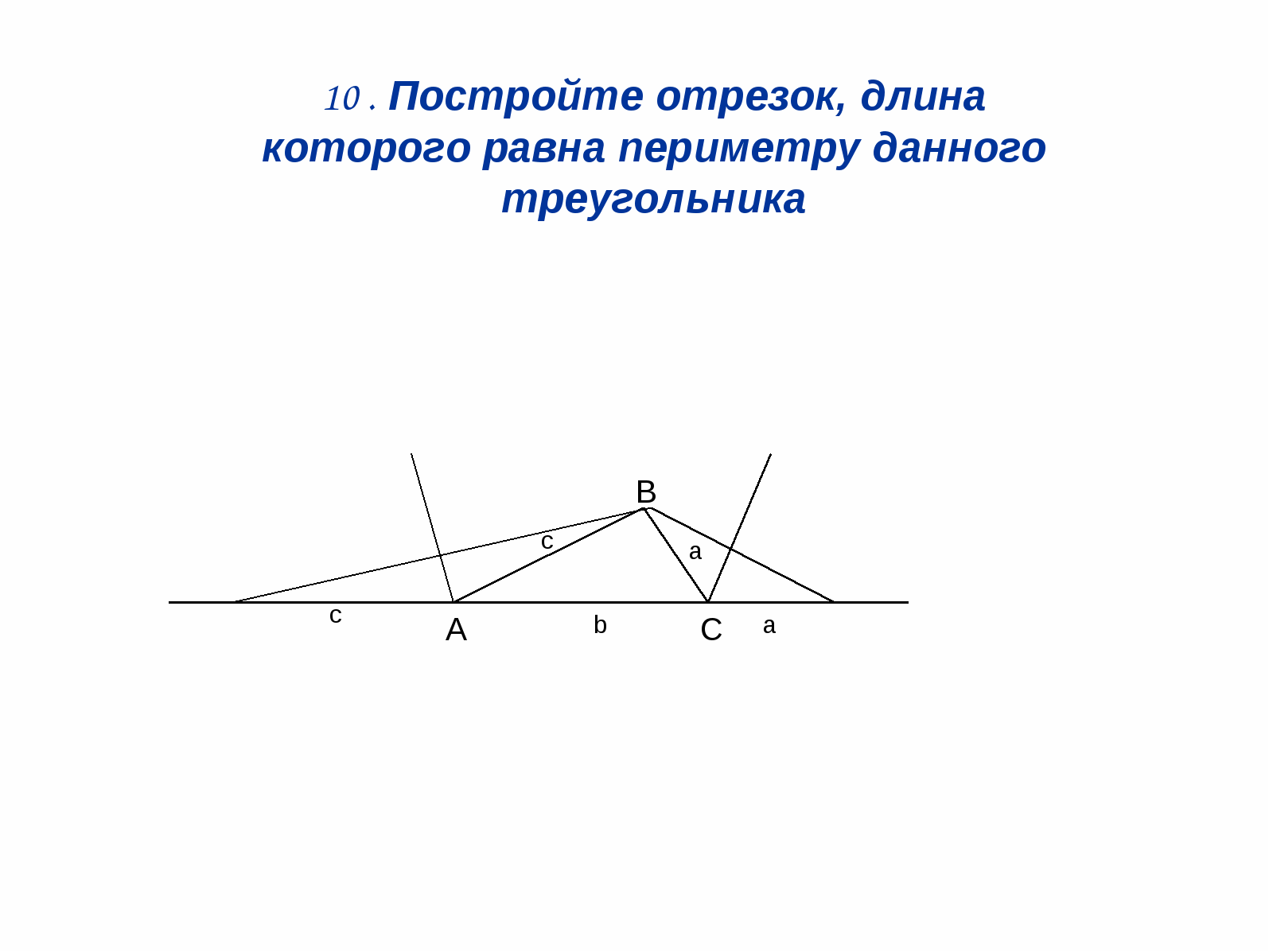equal to the perimeter of the given triangle.

Solution: (Fig. 12). Let's construct bisectors

two outer corners of the triangle, and then

3 peaks IN let's draw perpendiculars

to these bisectors.

DE = a + b + s

Fig.12

4. Given a segment of length a. Construct length segments 2a, 3a.

R solution: (Fig. 13)

1M N) || (AB) and (M 1 N 1 ) || (M N) || (M 2 N 2 ) –

Directly;

2) (CA) and (CB) through A and B.

The segments A 1 B 1 and A 2 B 2 are required.

Another solution to this problem can be

obtained from the solution to problem 7.

Rice. 13

5. Two segments are given on a straight line, the lengths of which are a and b . Construct segments whose lengths are equal to a + b , b - A, ( a + b )/2 and ( b - a )/2 .

Solution: and for a + b(Fig. 14, a)

Fig. 14, a

b) for ( a + b)/2 (Fig. 14, b)

1) (A 1 B 1) || (A 2 B 2) || (AB) – directly;

2) M Î (A 2 B 2), (MX) Ç (A 1 B 1 ) = N, (M H) Ç (A 1 B 1 ) = P;

3) (PY) Ç (A 2 B 2) = L, (LZ ) Ç (A 1 B 1 ) = O,

We get: N O = NP + P.O. =
.

Rice. 14, b

c) for b - A(Fig. 14, c)

Rice. 14,v

c) for ( b - a )/2 (Fig. 14, d)

Rice. 14,g

6
. Construct the center of this circle.

Solution : (Fig. 15) Let's draw a straight line AB,

intersecting the circle at points A and B;

Sun  AB, where C is the point of intersection

with a circle.

Through point C we draw parallel to AB

straight C D; WITHDintersects a circle

at the pointD.

ConnectingDwith B and A with C, we get

the desired point is the center of the circle. Rice. 15

Solution 2: (Fig. 16) Using a double-sided ruler, construct two parallel chordsAD AndB.C. . We get an isosceles trapezoidABCD. LetK AndP - points of intersection of linesA.C. AndBD , AB AndDC . Then straightP K passes through the midpoints of the bases of the trapezoid perpendicular to them, which means it passes through the center of the given circle. By similarly constructing another such straight line, we find the center of the circle.

Rice. 16

7. An arc of a circle is given. Construct the center of the circle

Solution . (Fig. 17) Mark three points A, B and C on this arc. Apply a ruler to the ends of the segment AB and trace its edges. We get two parallel lines. Changing the position of the ruler, we draw two more parallel lines. We get a rhombus (a parallelogram with equal heights). One of the diagonals of a rhombus is the perpendicular bisector to the segmentAB , since the diagonal of a rhombus lies on the perpendicular bisector to the other diagonal. Similarly, we construct the perpendicular bisector to the segmentA.C. . The intersection point of the constructed bisectors is the center of the desired circle.

Rice. 17

8. Given a segment AB, a non-parallel line l and a point M on it. Using one double-sided ruler, construct the points of intersection of straight line l with a circle of radius AB with center M.

Solution: (Fig.18)

Let's complete the triangleA.B.M. to parallelogramABNM . Let us construct the bisectors MT andMSangles betweenMNand straightl . Let's draw through the pointN lines parallel to these bisectors:NQ || MS, NR || M.T.. MT│ MSas bisectors of adjacent angles. Means,NQ │ MT, that is, in a triangleNMQthe bisector is the altitude, therefore the triangle is isosceles:MQ = MN. Likewise,M.R. = MN. PointsQAndRsought.

Rice. 18

9. Given a line l and a segment OA parallel to l. Using one double-sided ruler, construct the points of intersection of straight line l with a circle of radius OA with center O.

Solution: (Fig. 19,a)

Let's make a directl 1 , parallel to the lineO.A. and distant from it at a distancea . Let's take it on a straight linel arbitrary pointB . LetB 1 - point of intersection of linesO.B. Andl 1 . Let's draw through the pointB 1 straight, parallelAB ; this line intersects the lineO.A. at the pointA 1 . Let us now draw through the pointsO AndA 1 a pair of parallel lines, the distance between them isa (there can be two such pairs of lines); letX AndX 1 - points of intersection of a line passing through a pointO , with straight linesl Andl 1 . BecauseO.A. 1 = OX 1 and ∆O.A. 1 X 1 ∆ OAX , then OA = OX, pointX sought after.

Similarly, we construct the second point of intersection of the circle and the line - the pointY(Fig. 18, b).

Rice. 18,a

Rice. 18, b

I.6.Constructions with a one-sided ruler

Z
Here we consider a special case: let points P be given,Q, R 1 AndQ 1 . and they lie at the vertices of the trapezoid.

1. Divide segment P Q in half

Solution shown in Figure 19

Given points P,Q, R 1 AndQ 1 and parallel lines

RQ, R 1 Q 1 . Let's carry out RQ 1  QR 1 = B , RR 1  QQ 1 = A

Let's connect points A and B. AB RQ = F– middle

segment PQ.

Rice. 19

2. Double the segment R 1 Q 1.

R
decision shown in Figure 20. Let's build

pointF– the middle of the segment PQand connect it

WithQ 1. R 1 Q FQ 1 = M. Let's carry out RM. RM R 1 Q 1 = R

equalityRQand P 1 Q 1 follows from the similarity

triangles RMFAnd RMQ 1 ,

FMQAnd R 1 MQ 1 , and equalities PFAndFQ.

Rice. 20

3
. Construct a length segment n  R 1 Q 1 .

m – 1 equal segments PQ 2 , Q 2 Q 3, … Q m -1 Q m

Then we build (RR 1 ) AndQ m Q 1 and connect

their point of intersection A with points

Q 2 , Q 3, … Q m Receivedm -1 direct

divideR 1 Q 1 onm equal parts.

Form = 4 the solution is shown in Figure 22

Fig.22

I.7. Interchangeability of double-sided ruler with compass and ruler

Let us prove that a double-sided ruler is interchangeable with a compass and a ruler. To do this, we prove the following statements:

Statement 1: all constructions that can be done with a compass and ruler can be done with a double-sided ruler.

Since when constructing with a compass and a ruler, the ruler draws a line through two points, and the compass constructs a circle (finds a set of points equidistant from a given one), then all constructions with a compass and a ruler are reduced to constructing the intersection of two straight lines, two circles and a circle with a straight line.

The intersection of two straight lines can be constructed using a ruler.

The intersection of a circle and a straight line (Fig. 23):

Construction:Let the segment AB be given - the radius of the circle, a straight linel , the center of circle O, then:

1) We carry out the OS ||l , OS = AB.

2) We carry out the OS ||kand remote to a.

3) We carry outO.D., O.D. l = D; O.D. k) By corollary to Thales’ theorem

4) According to the law of transitivity of equalities

5) ConsiderOMQE. OMQEis a parallelogram, since OM ||EQand OE ||M.C.(the sides of the ruler are parallel). Let's prove that this is a rhombus.

5.1) ConductQZ O.C.AndQG ON, ThenQG = QZ = a.

5.2)  OMQ =  RQM(lying crosswise);  OS =ON, which was what needed to be proven.

Intersection of two circles: similar.

Statement 2: all constructions that can be done with a double-sided ruler can be done with a compass and straightedge.

To do this, we will perform the constructions standard for a double-sided ruler using a compass and a ruler.

1) A straight line using two points is easily constructed using a ruler.

2) Construction of a straight line parallel to a given one and removed from it at a given distance:

2.1) Let a straight line be givenkand length segmenta.

2.2) Construct an arbitrary straight lineb k, letk b= B.

2.3) Onbon both sides of the pointBon a straight linebset aside a piece of lengtha, let the pointsCAndD.

2.4) Through a pointCbuild a straight linec k.

2.5) Through a pointDbuild a straight lined k.

2.6) DirectcAndd-required, becauseB.C.AndBDequalaby construction and are equal to the distance between the straight linekand straight

3) Construction of straight lines parallel to each other and passing through two given points, and the distance between them is equal to the given segment:

3.1) Let points be givenAAndBand length segmenta.

3.2) Constructing a circle with a center at a pointAand radiusa.

3.3) Construct a tangent to a given circle through a pointB; there are two such tangents ifBlies outside the circle (ifAB> a), one ifBlies on the circle (ifAB= a), none ifBlies inside the circle (AB< a). This tangent is one of the lines we are looking for; it remains to pass through the pointAstraight line parallel to it.

3.4) Since one of the lines is perpendicular to the radius of the circle as a tangent, the second is also perpendicular to it (since they are parallel), therefore, the distance between them is equal to the radius, which by construction is equal toa, which is what was required to be obtained.

Thus, we have proven the interchangeability of a double-sided ruler and a compass and ruler.

Conclusion: A double-sided ruler is interchangeable with a compass and a ruler.

Conclusion

So, the question of the possibility of using one ruler to solve classical construction problems using a compass and a ruler has been considered and resolved. It turns out that construction problems can be solved using only a ruler with parallel edges. When solving more complex problems, one should further rely on the so-called basic constructions discussed in this work.

The presented material can be directly applied not only in mathematics lessons, in math circle classes, but also in practical activities.

List of used literature

Aliev A.V. Geometric constructions. Mathematics at school. 1978 No. 3

Glazer G.I. History of mathematics at school. M., Enlightenment. 1981.

Depman I.Ya. Behind the pages of a mathematics textbook. M.. Enlightenment. 1989.

Elensky Shch. In the footsteps of Pythagoras. M., Detgiz. 1961.

Encyclopedic dictionary of a young mathematician. M., Pedagogy. 1985

In construction tasks we will consider the construction of a geometric figure, which can be done using a ruler and compass.

Using a ruler you can:

arbitrary straight line;

an arbitrary straight line passing through a given point;

a straight line passing through two given points.

Using a compass, you can describe a circle of a given radius from a given center.

Using a compass you can plot a segment on a given line from a given point.

Let's consider the main construction tasks.

Task 1. Construct a triangle with given sides a, b, c (Fig. 1).

Solution. Using a ruler, draw an arbitrary straight line and take an arbitrary point B on it. Using a compass opening equal to a, we describe a circle with center B and radius a. Let C be the point of its intersection with the line. With a compass opening equal to c, we describe a circle from center B, and with a compass opening equal to b, we describe a circle from center C. Let A be the intersection point of these circles. Triangle ABC has sides equal to a, b, c.

Comment. In order for three straight segments to serve as sides of a triangle, it is necessary that the largest of them be less than the sum of the other two (and< b + с).

Task 2.

Solution. This angle with vertex A and the ray OM are shown in Figure 2.

Let us draw an arbitrary circle with its center at vertex A of the given angle. Let B and C be the points of intersection of the circle with the sides of the angle (Fig. 3, a). With radius AB we draw a circle with the center at point O - the starting point of this ray (Fig. 3, b). Let us denote the point of intersection of this circle with this ray as C 1 . Let us describe a circle with center C 1 and radius BC. Point B 1 of the intersection of two circles lies on the side of the desired angle. This follows from the equality Δ ABC = Δ OB 1 C 1 (the third sign of equality of triangles).

Task 3. Construct the bisector of this angle (Fig. 4).

Solution. From vertex A of a given angle, as from the center, we draw a circle of arbitrary radius. Let B and C be the points of its intersection with the sides of the angle. From points B and C we describe circles with the same radius. Let D be their intersection point, different from A. Ray AD bisects angle A. This follows from the equality Δ ABD = Δ ACD (the third criterion for the equality of triangles).

Task 4. Draw a perpendicular bisector to this segment (Fig. 5).

Solution. Using an arbitrary but identical compass opening (larger than 1/2 AB), we describe two arcs with centers at points A and B, which will intersect each other at some points C and D. The straight line CD will be the desired perpendicular. Indeed, as can be seen from the construction, each of the points C and D is equally distant from A and B; therefore, these points must lie on the perpendicular bisector to segment AB.

Task 5. Divide this segment in half. It is solved in the same way as problem 4 (see Fig. 5).

Task 6. Through a given point draw a line perpendicular to the given line.

Solution. There are two possible cases:

1) a given point O lies on a given straight line a (Fig. 6).

From point O we draw a circle with an arbitrary radius intersecting line a at points A and B. From points A and B we draw circles with the same radius. Let O 1 be the point of their intersection, different from O. We obtain OO 1 ⊥ AB. In fact, points O and O 1 are equidistant from the ends of the segment AB and, therefore, lie on the perpendicular bisector to this segment.

Municipal budgetary educational institution

secondary school No. 34 with in-depth study of individual subjects

MAN, physics and mathematics section

“Geometric constructions using compass and ruler”

Completed by: student of grade 7 “A”

Batishcheva Victoria

Head: Koltovskaya V.V.

Voronezh, 2013

3. Constructing an angle equal to the given one.

P Let's draw an arbitrary circle with a center at vertex A of a given angle (Fig. 3). Let B and C be the points of intersection of the circle with the sides of the angle. With radius AB we draw a circle with the center at point O, the starting point of this half-line. Let us denote the point of intersection of this circle with this half-line as C 1 . Let us describe a circle with center C 1 and Fig.3

radius of the aircraft. Point B 1 the intersection of the constructed circles in the indicated half-plane lies on the side of the desired angle.

6. Construction of perpendicular lines.

We draw a circle with an arbitrary radius r with a center at point O in Fig. 6. The circle intersects the line at points A and B.From points A and B we draw circles with radius AB. Let melancholy C be the point of intersection of these circles. We obtained points A and B in the first step, when constructing a circle with an arbitrary radius.

The desired straight line passes through points C and O.

Fig.6

Known Issues

1.Brahmagupta's problem

Construct an inscribed quadrilateral using its four sides. One solution uses the Apollonius circle.Let's solve Apollonius' problem using the analogy between a tricircle and a triangle. How we find a circle inscribed in a triangle: we construct the point of intersection of the bisectors, drop perpendiculars from it to the sides of the triangle, the bases of the perpendiculars (the points of intersection of the perpendicular with the side on which it is dropped) and give us three points lying on the desired circle. Draw a circle through these three points - the solution is ready. We will do the same with Apollonius' problem.

2. Apollonius' problem

Using a compass and ruler, construct a circle tangent to the three given circles. According to legend, the problem was formulated by Apollonius of Perga around 220 BC. e. in the book "Touch," which was lost, but was restored in 1600 by François Viète, the "Gallic Apollonius," as his contemporaries called him.

If none of the given circles lies inside the other, then this problem has 8 significantly different solutions.

Construction of regular polygons.

P

correct (or equilateral ) triangle - This regular polygonwith three sides, the first of the regular polygons. All sides of a regular triangle are equal to each other, and all the angles are 60°. To construct an equilateral triangle, you need to divide the circle into 3 equal parts. To do this, it is necessary to draw an arc of radius R of this circle from only one end of the diameter, we get the first and second divisions. The third division is at the opposite end of the diameter. By connecting these points, we get an equilateral triangle.

Regular hexagon Canconstruct using a compass and ruler. Belowthe construction method is giventhrough dividing the circle into 6 parts. We use the equality of the sides of a regular hexagon to the radius of the circumscribed circle. From the opposite ends of one of the diameters of the circle we describe arcs of radius R. The intersection points of these arcs with a given circle will divide it into 6 equal parts. By sequentially connecting the found points, a regular hexagon is obtained.

Construction of a regular pentagon.

P
a regular pentagon can beconstructed using a compass and ruler, or by fitting it into a givencircle, or construction based on a given side. This process is described by Euclidin his Elements about 300 BC. e.

Here is one method for constructing a regular pentagon in a given circle:

Construct a circle into which the pentagon will be inscribed and mark its center asO . (This is the green circle in the diagram on the right).

Select a point on the circleA , which will be one of the vertices of the pentagon. Construct a straight line throughO AndA .

Construct a line perpendicular to the lineO.A. , passing through the pointO . Designate one of its intersections with the circle as a pointB .

Plot a pointC in the middle betweenO AndB .

C through the pointA . Mark its intersection with the lineO.B. (inside the original circle) as a pointD .

Draw a circle with center atA through point D, mark the intersection of this circle with the original (green circle) as pointsE AndF .

Draw a circle with center atE through the pointA G .

Draw a circle with center atF through the pointA . Label its other intersection with the original circle as a pointH .

Construct a regular pentagonAEGHF .

Unsolvable problems

The following three construction tasks were set in antiquity:

Trisection of an angle - divide an arbitrary angle into three equal parts.

In other words, it is necessary to construct angle trisectors - rays dividing the angle into three equal parts. P. L. Wanzel proved in 1837 that the problem is solvable only when, for example, trisection is feasible for angles α = 360°/n, provided that the integer n is not divisible by 3. However, in the press from time to time (incorrect) methods for trisecting an angle with a compass and ruler are published.

Doubling the cube - classical ancient problem of constructing with a compass and ruler the edge of a cube, the volume of which is twice the volume of a given cube.

In modern notation, the problem is reduced to solving the equation. It all comes down to the problem of constructing a segment of length. P. Wantzel proved in 1837 that this problem could not be solved using a compass and straight edge.

Squaring a circle - a task consisting in finding a construction using a compass and a ruler of a square equal in area to the given circle.

As you know, with the help of a compass and a ruler you can perform all 4 arithmetic operations and extract the square root; it follows that squaring the circle is possible if and only if, using a finite number of such actions, it is possible to construct a segment of length π. Thus, the unsolvability of this problem follows from the non-algebraic nature (transcendence) of the number π, which was proved in 1882 by Lindemann.

Another well-known problem that cannot be solved using a compass and ruler isconstructing a triangle using three given bisector lengths .

Moreover, this problem remains unsolvable even in the presence of a trisector.

It was only in the 19th century that it was proven that all three problems were unsolvable using only a compass and straightedge. The question of the possibility of construction is completely resolved by algebraic methods based on Galois theory.

DID YOU KNOW THAT...

(from the history of geometric constructions)

Once upon a time, a mystical meaning was invested in the construction of regular polygons.

Thus, the Pythagoreans, followers of the religious and philosophical teaching founded by Pythagoras, and who lived in ancient Greece (V I-I Vcenturies BC BC), adopted as a sign of their union a star-shaped polygon formed by the diagonals of a regular pentagon.

The rules for the strict geometric construction of some regular polygons are set out in the book “Elements” by the ancient Greek mathematician Euclid, who lived inIIIV. BC. To carry out these constructions, Euclid proposed using only a ruler and a compass, which at that time did not have a hinged device for connecting the legs (such a limitation in instruments was an immutable requirement of ancient mathematics).

Regular polygons were widely used in ancient astronomy. If Euclid was interested in the construction of these figures from the point of view of mathematics, then for the ancient Greek astronomer Claudius Ptolemy (about 90 - 160 AD) it turned out to be necessary as an auxiliary tool in solving astronomical problems. So, in the 1st book of the Almagests, the entire tenth chapter is devoted to the construction of regular pentagons and decagons.

However, in addition to purely scientific works, the construction of regular polygons was an integral part of books for builders, craftsmen, and artists. The ability to depict these figures has long been required in architecture, jewelry, and fine arts.

The “Ten Books on Architecture” of the Roman architect Vitruvius (who lived approximately 63-14 BC) says that the city walls should have the form of a regular polygon in plan, and the towers of the fortress “should be made round or polygonal, for a quadrangle rather destroyed by siege weapons.”

The layout of cities was of great interest to Vitruvius, who believed that it was necessary to plan the streets so that the main winds did not blow along them. It was assumed that there were eight such winds and that they blew in certain directions.

During the Renaissance, the construction of regular polygons, and in particular the pentagon, was not a simple mathematical game, but was a necessary prerequisite for the construction of fortresses.

The regular hexagon was the subject of a special study by the great German astronomer and mathematician Johannes Kepler (1571-1630), which he talks about in his book “New Year's Gift, or Hexagonal Snowflakes.” Discussing the reasons why snowflakes have a hexagonal shape, he notes, in particular, the following: “... a plane can be covered without gaps only with the following figures: equilateral triangles, squares and regular hexagons. Among these figures, the regular hexagon covers the largest area."

One of the most famous scientists involved in geometric constructions was the great German artist and mathematician Albrecht Durer (1471 -1528), who dedicated a significant part of his book “Manuals...” to them. He proposed rules for constructing regular polygons with 3, 4, 5... 16 sides. The methods for dividing a circle proposed by Dürer are not universal; an individual technique is used in each specific case.

Dürer used methods for constructing regular polygons in artistic practice, for example, when creating various kinds of ornaments and patterns for parquet. He sketched such patterns during a trip to the Netherlands, where parquet floors were found in many homes.

Dürer composed ornaments from regular polygons, which are connected into rings (rings of six equilateral triangles, four quadrangles, three or six hexagons, fourteen heptagons, four octagons).

Conclusion

So,geometric constructions is a method of solving a problem in which the answer is obtained graphically. Constructions are carried out using drawing tools with maximum precision and accuracy of work, since the correctness of the solution depends on this.

Thanks to this work, I became acquainted with the history of the origin of the compass, became more familiar with the rules for performing geometric constructions, gained new knowledge and applied it in practice.
Solving problems involving construction with compasses and a ruler is a useful pastime that allows you to take a fresh look at the known properties of geometric figures and their elements.This paper discusses the most pressing problems associated with geometric constructions using compasses and rulers. The main problems are considered and their solutions are given. The given problems are of significant practical interest, consolidate acquired knowledge in geometry and can be used for practical work.
Thus, the goal of the work has been achieved, the assigned tasks have been completed.

Src="https://present5.com/presentation/3/178794035_430371946.pdf-img/178794035_430371946.pdf-1.jpg" alt=">Construction using a ruler and compass Geometry">!}

Src="https://present5.com/presentation/3/178794035_430371946.pdf-img/178794035_430371946.pdf-2.jpg" alt="> Construct a segment equal to the given Ú Problem A B"> Построить отрезок равный данному Ú Задача А В На данном луче от его начала С отложить отрезок, равный данному Ú Решение 1. Изобразим фигуры, данные в D условии задачи: луч ОС и отрезок АВ О 2. Затем циркулем построим окружность радиуса АВ и с центром О. 3. Эта окружность пересечёт луч ОС в некой точке D. Отрезок OD – искомый.!}

Src="https://present5.com/presentation/3/178794035_430371946.pdf-img/178794035_430371946.pdf-3.jpg" alt="> Constructing an angle equal to a given one Consider triangles"> Построение угла равного данному Рассмотрим треугольники Ú АВС и ОDE. Задача В Отрезки АВ и АС являются равный Отложить от данного луча угол, данному Ú радиусами окружности с Решение 1. центром А, савершиной А и луч и ОЕ Построим угол отрезки OD ОМ А С 2. – радиусами окружности с Проведем окружность произвольного центром О. Таквершине А данного радиуса с центром в как по угла. 3. построениюпересекает стороны Эта окружность эти окружности имеют равные радиусы, то угла в точках В и С. 4. АВ=OD, AC=OE. Также же Затем проведём окружность того по Е радиуса с центром в начале данного построению ВС=DE. М луча ОМ. О D Следовательно, треугольники 5. Она пересекает луч в точке D. 6. равны по построим окружность с После этого 3 сторонам. Поэтому центром D, радиус которой равен ВС 7. угол DOEс= углу BAC. Т. е. Окружности центрами О и D построенный угол МОЕ равен пересекаются в двух точках. Одну из углу А. буквой Е них назовём 8. Докажем, что угол МОЕ - искомый!}

Src="https://present5.com/presentation/3/178794035_430371946.pdf-img/178794035_430371946.pdf-4.jpg" alt="> Constructing the bisector of an angle Problem Ú"> Построение биссектрисы угла Задача Ú Рассмотрим треугольники Ú АСЕ и АВЕ. биссектрису угла Построить Они равны по Ú трём сторонам. АЕ – общая, Решение Е 1. АС и АВ равны как угол ВАС Изобразим данный радиусы 2. одной и тойокружность Проведём же окружности, В СЕ = ВЕ по построению. произвольного радиуса с С Ú Изцентром А. Она пересечёт равенства треугольников следует, что угол САЕ В и С стороны угла в точках = углу 3. ВАЕ, т. е. луч АЕдве Затем проведём – окружности одинакового биссектриса данного угла. А радиуса ВС с центрами в точках В и С 4. Докажем, что луч АЕ – биссектриса угла ВАС!}

Src="https://present5.com/presentation/3/178794035_430371946.pdf-img/178794035_430371946.pdf-5.jpg" alt="> Construction of perpendicular lines Ú Problem Given a line"> Построение перпендикулярных прямых Ú Задача Даны прямая и точка на ней. Построить прямую, проходящую через данную точку Р и перпендикулярную данной прямой. Ú Решение 1. Построим прямую а и точку М, принадлежащую этой прямой. 2. На лучах прямой а, исходящих из точки М, отложим равные отрезки МА и МВ. М а Затем построим две окружности с центрами А и В радиуса АВ. Они пересекутся в двух точках: P и Q. А B 3. Проведём прямую через точку М и одну из этих точек, например прямую МР, и докажем, что эта прямая искомая, т. Е. что она перпендикулярна к данной прямой. 4. В самом деле, так как медиана РМ равнобедренного треугольника РАВ Q является также высотой, то РМ перпендикулярна а.!}

Src="https://present5.com/presentation/3/178794035_430371946.pdf-img/178794035_430371946.pdf-6.jpg" alt="> Constructing the midpoint of a segment Task Ú Construct the midpoint of a given"> Построение середины отрезка Задача Ú Построить середину данного отрезка Ú Решение Р 1. Пусть АВ – данный отрезок. 2. Построим две окружности с 21 центрами А и В радиуса АВ. Они пересекаются в точках Р и Q. О 3. Проведём прямую РQ. Точка О пересечения этой прямой с А B отрезком АВ и есть искомая середина отрезка АВ 4. В самом деле, треугольники АРQ и ВРQ равны по трём сторонам, поэтому угол 1 = Q углу 2 5. Следовательно отрезок РО – биссектриса равнобедренного треугольника АРВ, а значит, и медиана, т. Е. точка О – середина отрезка АВ.!}