What does it mean to evaluate an integral. Edit] Estimating the accuracy of computing a definite integral

definite integral from continuous function f(x) on the finite interval [ a, b] (where ) is the increment of some of its antiderivatives on this segment. (In general, understanding will be noticeably easier if you repeat the topic of the indefinite integral) In this case, the notation

As can be seen in the graphs below (the increment of the antiderivative function is indicated by ), The definite integral can be either positive or negative number (It is calculated as the difference between the value of the antiderivative in the upper limit and its value in the lower limit, i.e. as F(b) - F(a)).

Numbers a and b are called the lower and upper limits of integration, respectively, and the interval [ a, b] is the segment of integration.

Thus, if F(x) is some antiderivative function for f(x), then, according to the definition,

(38)

Equality (38) is called Newton-Leibniz formula . Difference F(b) – F(a) is briefly written like this:

Therefore, the Newton-Leibniz formula will be written as follows:

(39)

Let us prove that the definite integral does not depend on which antiderivative of the integrand is taken when calculating it. Let F(x) and F( X) are arbitrary antiderivatives of the integrand. Since these are antiderivatives of the same function, they differ by a constant term: Ф( X) = F(x) + C. That's why

Thus, it is established that on the segment [ a, b] increments of all antiderivatives of the function f(x) match.

Thus, to calculate the definite integral, it is necessary to find any antiderivative of the integrand, i.e. must first be found indefinite integral. Constant FROM excluded from subsequent calculations. Then the Newton-Leibniz formula is applied: the value of the upper limit is substituted into the antiderivative function b , further - the value of the lower limit a and calculate the difference F(b) - F(a) . The resulting number will be a definite integral..

At a = b accepted by definition

Example 1

Solution. Let's find the indefinite integral first:

Applying the Newton-Leibniz formula to the antiderivative

(at FROM= 0), we get

However, when calculating a definite integral, it is better not to find the antiderivative separately, but immediately write the integral in the form (39).

Example 2 Calculate a definite integral

Solution. Using the formula

Properties of the Definite Integral

Theorem 2.The value of the definite integral does not depend on the designation of the integration variable, i.e.

(40)

Let F(x) is antiderivative for f(x). For f(t) the antiderivative is the same function F(t), in which the independent variable is denoted differently. Consequently,

Based on formula (39), the last equality means the equality of the integrals

Theorem 3.The constant factor can be taken out of the sign of a definite integral, i.e.

(41)

Theorem 4.Definite integral from the algebraic sum finite number functions is algebraic sum definite integrals of these functions, i.e.

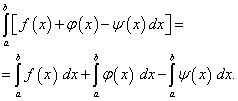(42)

Theorem 5.If the segment of integration is divided into parts, then the definite integral over the entire segment is equal to the sum of definite integrals over its parts, i.e. if

(43)

Theorem 6.When rearranging the limits of integration absolute value of a definite integral does not change, but only its sign changes, i.e.

(44)

Theorem 7(mean value theorem). Definite integral is equal to the product the length of the segment of integration by the value of the integrand at some point inside it, i.e.

(45)

Theorem 8.If the upper integration limit is greater than the lower one and the integrand is non-negative (positive), then the definite integral is also non-negative (positive), i.e. if

Theorem 9.If the upper limit of integration is greater than the lower limit and the functions and are continuous, then the inequality

can be integrated term by term, i.e.

(46)

The properties of the definite integral allow us to simplify the direct calculation of integrals.

Example 5 Calculate a definite integral

Using Theorems 4 and 3, and when finding antiderivatives - tabular integrals (7) and (6), we obtain

Definite integral with variable upper limit

Let f(x) is continuous on the interval [ a, b] function, and F(x) is its prototype. Consider the definite integral

(47)

and through t the integration variable is denoted so as not to confuse it with the upper bound. When it changes X the definite integral (47) also changes, i.e., it is a function of the upper limit of integration X, which we denote by F(X), i.e.

(48)

Let us prove that the function F(X) is antiderivative for f(x) = f(t). Indeed, differentiating F(X), we get

because F(x) is antiderivative for f(x), a F(a) is a constant value.

Function F(X) - one of an infinite number antiderivatives for f(x), namely the one that x = a goes to zero. This statement is obtained if in equality (48) we put x = a and use Theorem 1 of the previous section.

Calculation of definite integrals by the method of integration by parts and the method of change of variable

where, by definition, F(x) is antiderivative for f(x). If in the integrand we make the change of variable

then, in accordance with formula (16), we can write

In this expression

antiderivative function for

Indeed, its derivative, according to the rule of differentiation of a complex function, is equal to

Let α and β be the values ​​of the variable t, for which the function

takes respectively the values a and b, i.e.

But, according to the Newton-Leibniz formula, the difference F(b) – F(a) there is

Trapezoidal method

Main article:Trapezoidal method

If the function on each of the partial segments is approximated by a straight line passing through the final values, then we obtain the trapezoid method.

The area of ​​the trapezoid on each segment:

Approximation error on each segment:

where

Full formula trapezoids in the case of dividing the entire integration interval into segments the same length :

where

Trapezoidal formula error:

where

Simpson method.

Integrand f(x) is replaced by an interpolation polynomial of the second degree P(x)– a parabola passing through three nodes, for example, as shown in the figure ((1) is a function, (2) is a polynomial).

Consider two steps of integration ( h= const = x i+1 – x i), that is, three nodes x0, x1, x2, through which we draw a parabola, using Newton's equation:

Let z = x - x0,
then

Now, using the obtained relation, we calculate the integral over this interval:

.
For uniform grid and even number of steps n Simpson's formula becomes:

Here , a under the assumption that the fourth derivative of the integrand is continuous.

[edit] Increasing Accuracy

Approximation of a function by one polynomial over the entire interval of integration, as a rule, leads to big mistake in estimating the value of the integral.

To reduce the error, the integration segment is divided into parts and applied numerical method to evaluate the integral on each of them.

As the number of partitions tends to infinity, the estimate of the integral tends to its true value for analytic functions for any numerical method.

The above methods allow for a simple procedure of halving the step, while at each step it is required to calculate the function values ​​only at newly added nodes. The Runge rule is used to estimate the calculation error.

Application of Runge's rule

edit] Estimating the accuracy of computing a definite integral

The integral is calculated using the chosen formula (rectangles, trapezoids, Simpson's parabolas) with the number of steps equal to n, and then with the number of steps equal to 2n. The error in calculating the value of the integral with the number of steps equal to 2n is determined by the Runge formula:
, for the formulas of rectangles and trapezoids, and for the Simpson formula.
Thus, the integral is calculated for consecutive values number of steps, where n 0 is the initial number of steps. The calculation process ends when the next value N will satisfy the condition , where ε is the specified accuracy.

Features of the behavior of the error.

It would seem that why analyze different methods of integration if we can achieve high accuracy by simply reducing the value of the integration step. However, consider the graph of the behavior of the a posteriori error R results of numerical calculation depending on and from the number n interval partitions (that is, at step . In section (1), the error decreases due to a decrease in step h. But in section (2), the computational error begins to dominate, accumulating as a result of numerous arithmetic operations. Thus, each method has its own Rmin, which depends on many factors, but primarily on the a priori value of the error of the method R.

Refinement formula of Romberg.

The Romberg method consists in successive refinement of the value of the integral with a multiple increase in the number of partitions. The formula of trapezoids with a uniform step can be taken as the base h.
Denote the integral with the number of partitions n= 1 as .
Decreasing the step by half, we get .
If we successively decrease the step by 2n times, we get recurrence relation for calculation .

Theorem. If the function f(x) integrable on the interval [ a, b], where a< b , and for all x ∈ the inequality

Using the inequalities from the theorem, one can estimate the definite integral, i.e. indicate the boundaries between which its meaning is enclosed. These inequalities express an estimate for a definite integral.

Theorem [Mean value theorem]. If the function f(x) integrable on the interval [ a, b] and for all x ∈ the inequalities m ≤ f(x) ≤ M, then

where m ≤ μ ≤ M.

Comment. In the case where the function f(x) continuous on the segment [ a, b], the equality from the theorem takes the form

where c ∈. Number μ=f(c) defined by this formula is called average functions f(x) on the segment [ a, b]. This equality has the following geometric sense : square curvilinear trapezoid bounded by a continuous line y=f(x) (f(x) ≤ 0) is equal to the area of ​​a rectangle with the same base and a height equal to the ordinate of some point on this line.

Existence of an antiderivative for a continuous function

First, we introduce the concept of an integral with a variable upper limit.

Let the function f(x) integrable on the interval [ a, b]. Then whatever the number x from [ a, b], function f(x) integrable on the interval [ a, b]. Therefore, on the segment [ a, b] function defined

which is called an integral with a variable upper limit.

Theorem. If the integrand is continuous on the interval [ a, b], then the derivative of a definite integral with a variable upper limit exists and is equal to the value of the integrand for this limit, i.e.

Consequence. The definite integral with a variable upper limit is one of the antiderivatives for a continuous integrand. In other words, for any function continuous on an interval, there exists an antiderivative.

Remark 1. Note that if the function f(x) integrable on the interval [ a, b], then the integral with a variable upper limit is a continuous function of the upper limit on this segment. Indeed, from St. 2 and the mean value theorem we have

Remark 2. The integral with a variable upper limit of integration is used in the definition of many new functions, for example, . These functions are not elementary; as already noted, the antiderivatives of the indicated integrands cannot be expressed in terms of elementary functions.

Basic integration rules

Newton-Leibniz formula

Since any two antiderivative functions f(x) differ by a constant, then, according to the previous theorem, it can be argued that any antiderivative Φ(x) continuous on the segment [ a, b] functions f(x) has the form

where C is some constant.

Putting in this formula x=a and x=b, using St.1 definite integrals, we find

From these equalities follows the relation

which is called Newton-Leibniz formula.

Thus we have proved the following theorem:

Theorem. The definite integral of a continuous function is equal to the difference between the values ​​of any of its antiderivatives for the upper and lower integration limits.

The Newton-Leibniz formula can be rewritten as

Change of variable in a definite integral

Theorem. If a

• function f(x) continuous on the segment [ a, b];
• line segment [ a, b] is the set of function values φ(t) defined on the interval α ≤ t ≤ β and having a continuous derivative on it;
• φ(α)=a, φ(β)=b

then the formula is valid

Integration by parts formula

Theorem. If functions u=u(x), v=v(x) have continuous derivatives on the interval [ a, b], then the formula

Mean theorem. If f(x) is continuous on the segment , then there exists a point such that . Doc. A function that is continuous on a segment takes its smallest m and largest M values ​​on this segment. Then . Number is between the minimum and maximum values ​​of the function on the interval. One of the properties of a function that is continuous on an interval is that this function takes on any value between m and M. Thus, there is a point such that . This property has a simple geometric interpretation: if is continuous on the segment , then there is a point such that the area of ​​the curvilinear trapezoid ABCD is equal to the area of ​​the rectangle with base and height f(c) (highlighted in the figure).

7. Integral with a variable upper limit. Its continuity and differentiability.

Consider a function f (x) that is Riemann-integrable on the interval . Since it is integrable on , then it is also integrable on ∀x ∈ . Then for each x ∈ the expression makes sense, and for each x it is equal to some number.

Thus, each x ∈ is associated with some number ,

those. function is given:

(3.1)

Definition:

The function F (x) given in (3.1), as well as the expression itself, is called

integral with a variable upper limit. It is defined on the entire segment

integrability of the function f (x).

Condition: f (t) is continuous on , and the function F (x) is given by formula (3.1).

Statement: The function F(x) is differentiable on , and F (x) = f (x).

(At a it is right differentiable, and at b it is left differentiable.)

Proof:

Since for a function of one variable F (x) differentiability is equivalent to the existence of a derivative at all points (at point a on the right, and at point b on the left), then we will find the derivative F (x). Consider the difference

In this way,

moreover, the point ξ lies on the segment (or if ∆x< 0).

Now recall that the derivative of the function F(x) at a given point x ∈ is equal to the limit of the difference relation: . From equality we have:

,

Letting ∆x → 0 now, on the left side of this equality we obtain F’(x), and on the right

Recall the definition of the continuity of the function f (t) at the point x:

Let x1 be equal to ξ in this definition. Since ξ ∈ (ξ ∈ ) and

∆x → 0, then |x − ξ| → 0, and by the definition of continuity, f (ξ) → f (x). Hence we have:

F'(x) = f(x).

Consequence:

Condition: f (x) is continuous on .

Statement: Any antiderivative of the function f (x) has the form

where C ∈ R is some constant.

Proof. By Theorem 3.1, the function is a prototype for f(x). Suppose that G(x) is another antiderivative f (x). Then G'(x) = f(x) and for the function F(x) − G(x) we have: (F (x) + G(x))' = F'(x)−G'(x) = f (x)−f(x) ≡ 0. Hence, the derivative of the function F (x)−G(x)

is equal to zero, therefore, this function is a constant: F(x) − G(x) = const.

8. Newton-Leibniz formula for a definite integral.

Theorem:

Condition: f(t) is continuous on , and F(x) is its any antiderivative.

Statement:

Proof: Consider some antiderivative F (x) of the function f (x). According to the Corollary from the Theorem “On the Differentiability of an Integral with a Variable Upper Limit” (see the previous question), it has the form . From here

=> c= F(a) , and

We move F(a) in the last equality to the left-hand side, re-denoting integration variable again through x and get the Newton-Leibniz formula:

Applied value mean value theorems consists in the possibility of obtaining a qualitative estimate of the value of a certain integral without calculating it. We formulate : if the function is continuous on the interval , then inside this interval there is such a point that .

This formula is quite suitable for a rough estimate of the integral of a complex or cumbersome function. The only moment that makes the formula approximate , is a necessity self-selection points . If we take the simplest path - the middle of the integration interval (as suggested in a number of textbooks), then the error can be quite significant. For more exact result recommend carry out the calculation in the following sequence:

Construct a function graph on the interval ;

Draw the upper border of the rectangle in such a way that the cut off parts of the graph of the function are approximately equal in area (this is exactly how it is shown in the above figure - two curvilinear triangles are almost the same);

Determine from figure ;

Use the mean value theorem.

As an example, let's calculate a simple integral:

Exact value ;

For the middle of the interval we will also obtain an approximate value , i.e. clearly inaccurate result;

Having built a graph with drawing the upper side of the rectangle in accordance with the recommendations, we get , from where and the approximate value of . Quite satisfactory result, the error is 0.75%.

Trapezoidal formula

The accuracy of calculations using the mean value theorem essentially depends, as was shown, on visual purpose point chart. Indeed, by choosing, in the same example, points or , you can get other values ​​of the integral, and the error may increase. Subjective factors, the scale of the graph and the quality of the drawing greatly affect the result. it unacceptably in critical calculations, so the mean value theorem applies only to fast quality integral estimates.

In this section, we will consider one of the most popular methods of approximate integration - trapezoid formula . The basic idea of ​​constructing this formula comes from the fact that the curve can be approximately replaced by a broken line, as shown in the figure.

Let us assume, for definiteness (and in accordance with the figure), that the integration interval is divided into equal (this is optional, but very convenient) parts. The length of each of these parts is calculated by the formula and is called step . The abscissas of the split points, if specified, are determined by the formula , where . It is easy to calculate ordinates from known abscissas. In this way,

This is the trapezoid formula for the case. Note that the first term in brackets is the half-sum of the initial and final ordinates, to which all intermediate ordinates are added. For an arbitrary number of partitions of the integration interval general formula trapezoid looks like: quadrature formulas: rectangles, simpson, gauss, etc. They are built on the same idea of ​​representing a curvilinear trapezoid by elementary areas various shapes, therefore, after mastering the trapezoid formula, it will not be difficult to understand similar formulas. Many formulas are not as simple as the trapezoid formula, but allow you to get a high accuracy result with a small number of partitions.

With the help of the trapezoid formula (or similar ones), it is possible to calculate, with the accuracy necessary in practice, both "non-taking" integrals and integrals of complex or cumbersome functions.