Rule of lopital wording. L'Hopital's rule and disclosure of uncertainties

The rule says that if the functions f(x) and g(x) have the following set of conditions:

then there is . Moreover, the theorem is also true for other bases (the proof will be given for the indicated one).

Story

A method for disclosing this kind of uncertainty was published by Lopital in his work "Analysis of infinitesimals", published in the year. In the preface to this work, Lopital points out that he used the discoveries of Leibniz and the Bernoulli brothers without any hesitation and "has nothing against them showing their copyright to whatever they want." Johann Bernoulli laid claim to L'Hospital's entire work, and in particular, after L'Hospital's death, he published a work under the remarkable title "Improvement of my method published in Infinitesimal Analysis for determining the value of a fraction, the numerator and denominator of which sometimes disappear", .

Proof

The ratio of infinitesimals

Let us prove the theorem for the case when the limits of the functions are equal to zero (the so-called uncertainty of the form ).

Since we are looking at functions f and g only in the right punctured semineighbourhood of the point a, we can continuously redefine them at this point: let f(a) = g(a) = 0 . Let's take some x from the semineighborhood under consideration and apply the Cauchy theorem to the segment. By this theorem, we get:

but f(a) = g(a) = 0 , That's why .

src="/pictures/wiki/files/56/85e2b8bb13d6fb1ddcf88e22a4bb6ef2.png" border="0"> for end limit and src="/pictures/wiki/files/101/e8b2f2b8861947c8728d4d1be40366d4.png" border="0"> for infinity ,

which is the definition of the limit of the ratio of functions.

The ratio of infinitely large

Let us prove the theorem for uncertainties of the form .

Let, for starters, the limit of the ratio of derivatives be finite and equal to A. Then, while striving x to a on the right, this relation can be written as A+ α , where α - (1). Let's write this condition:

Let's fix t from the segment and apply the Cauchy theorem to all x from the segment:

, which can lead to next kind:

For x, close enough to a, the expression makes sense; limit of the first factor of the right hand side equal to one(as f(t) and g(t) are constants , and f(x) and g(x) tend to infinity). Hence, this factor is equal to 1 + β, where β is an infinitesimal function as x to a on right. We write out the definition of this fact, using the same value as in the definition for α :

We have found that the ratio of functions can be represented in the form (1 + β)( A+ α) , and . For any given one, one can find such that the modulus of the difference between the ratios of the functions and A was less, which means that the limit of the ratio of functions is really equal to A .

If the limit A is infinite (let's say it is equal to plus infinity), then

(x))(g"(x))>2M)" src="/pictures/wiki/files/101/e46c5113c49712376d1c357b5b202a65.png" border="0">.

In the definition of β we will take ; the first factor of the right side will be greater than 1/2 when x, close enough to a, and then src="/pictures/wiki/files/50/2f7ced4a9b4b06f7b9085e982250dbcf.png" border="0">.

For other bases, the proofs are similar to those given.

Examples

(Only if the numerator and denominator BOTH tend to either 0 ; or ; or .)

Wikimedia Foundation. 2010 .

See what the "L'Hopital rule" is in other dictionaries:

Historically incorrect name for one of the basic rules for disclosure of uncertainties. L. p. was found by I. Bernoulli and reported by him to G. L'Hopital (See L'Hopital), who published this rule in 1696. See Indefinite expressions ... Great Soviet Encyclopedia

Disclosure of uncertainties of the form by reducing the limit of the ratio of functions to the limit of the ratio of the derivatives of the functions under consideration. So, for the case when real functions f and g are defined in a punctured right-hand neighborhood of a numerical point ... ... Mathematical Encyclopedia

Bernoulli L'Hospital's rule is a method for finding the limits of functions, revealing uncertainties of the form u. The theorem justifying the method states that under certain conditions the limit of the ratio of functions is equal to the limit of the ratio of their derivatives. ... ... Wikipedia

In mathematical analysis, L'Hopital's rule is a method for finding the limits of functions, revealing uncertainties of the form 0 / 0 and. The theorem justifying the method states that under certain conditions the limit of the ratio of functions is equal to the limit ... ... Wikipedia

Dependences of coordinates on time when moving material point in plane

Determine module speed (

A. The modulus of the velocity of a material point from time is expressed by the formula:

B. . The module of acceleration of a material point from time is expressed by the formula:

These equations describe the motion of a material point with constant acceleration

The satellite revolves around the earth in a circular orbit at a height

A satellite moving in a circular orbit is subject to the force of gravity

This formula can be simplified as follows. On a body weight

Thus, line speed satellite is

and the angular velocity

Both balls considered in the problem form a closed system and in the case elastic shock both the momentum of the system and the mechanical (kinetic) energy are conserved. Let us write down both conservation laws (taking into account the immobility of the second ball before the impact):

Thus, the incident (first) ball as a result of the impact reduced its speed from 1.05 m/s to 0.45 m/s, although it continued to move in the same direction, and the previously stationary (second) ball acquired a speed equal to 1, 5 m/s and now both balls are moving in the same straight line and in the same direction.

Since the mass of the gas in the balloon changes, the initial and final states of the gas in the balloon cannot be related either by the Boyle-Mariotte law or by the Charles law. If the gas in the balloon changes, the initial and final states of the gas in the balloon cannot be related by the Boyle-Mariotte law. each state write the Mendeleev-Clapeyron equation

How to find the limit of a function without using the lopital rule

System version:
7.47 (16.04.2018)

General news:
13.04.2018, 10:33

Last question:
26.07.2018, 15:23

Last reply:
27.07.2018, 13:48

SECTION Mathematics

Consultations and problem solving in algebra, geometry, analysis, discrete mathematics.

The best experts in this section

Hello! I'm having trouble with this question:

Find the limit of a function without using L'Hopital's rule

lim (2x+3) [ ln (x+2) - ln x ] (under lim it is written "x tends to infinity")

There were several examples of limits in the assignment, but this one baffled. I don't know how to solve it. Maybe somehow use the second wonderful limit, but how (only this thought comes to mind)?

Let me just ask in the same question whether such a statement of the problem takes place (if it does, I will post it later as a paid question): Applying the Taylor formula with a remainder term in the Lagrange form to the function, calculate the value with an accuracy of 0.001; a = 0.29.
Here I do not understand what function? It is not set (?), the task sounds exactly as I wrote it down. Maybe you can take the function yourself, but which one?

Status: Consultation closed

Hello Aleksandrkib!
It is the 2nd one that you need to use! To start, let's simplify:
lim (2x+3) [ ln (x+2) - ln x ] = lim (2x+3) ln ((x+2)/x) = lim (2x+3) ln (1+2/x) = lim ln ((1+2/x)^(2x+3)) = lim ln ((1+2/x)^2x)+lim ln ((1+2/x)^3) [the second limit is zero , since 2/x tends to zero and ln 1 = 0]
Let's make the change y = x/2, then lim ln ((1+2/x)^2x) = 4 lim ln ((1+1/y)^y) = 4 * ln e =4. Answer: 4.

There must be some function.

Send messages
moderators can
only members of the portal.
LOGIN TO THE PORTAL »
registration "

L'Hopital's rule: theory and examples of solutions

L'Hopital's rule and disclosure of uncertainties

The disclosure of uncertainties of the form 0/0 or ∞/∞ and some other uncertainties that arise when calculating the limit of the ratio of two infinitesimal or infinitely large functions is greatly simplified using L'Hopital's rule (actually two rules and comments on them).

essence rules of L'Hospital is that in the case when the calculation of the limit of the ratio of two infinitesimal or infinitely large functions gives uncertainties of the form 0/0 or ∞/∞, the limit of the ratio of two functions can be replaced by the limit of the ratio of their derivatives and, thus, a certain result can be obtained.

Let's move on to the formulation of L'Hopital's rules.

L'Hopital's Rule for the Case of the Limit of Two Infinitely Small Values. If functions f(x) and g(x a a, and in this neighborhood g‘(x a equal to each other and equal to zero

(),

then the limit of the ratio of these functions is equal to the limit of the ratio of their derivatives

().

L'Hôpital's rule for the case of the limit of two infinitely large quantities. If functions f(x) and g(x) are differentiable in some neighborhood of the point a, with the possible exception of the point a, and in this neighborhood g‘(x)≠0 and if and if the limits of these functions as x tends to the value of the function at the point a equal to each other and equal to infinity

(),

In other words, for uncertainties of the form 0/0 or ∞/∞, the limit of the ratio of two functions is equal to the limit of the ratio of their derivatives, if the latter exists (finite or infinite).

Remarks.

1. L'Hopital's rules are also applicable when the functions f(x) and g(x) are not defined at x = a.

2. If, when calculating the limit of the ratio of derivatives of functions f(x) and g(x) we again come to an uncertainty of the form 0/0 or ∞/∞, then L'Hopital's rules should be applied repeatedly (at least twice).

3. L'Hopital's rules are also applicable when the argument of functions (x) tends not to final number a, and to infinity ( x → ∞).

Uncertainties of other types can also be reduced to uncertainties of the types 0/0 and ∞/∞.

Disclosure of uncertainties of the types "zero divided by zero" and "infinity divided by infinity"

Example 1

x=2 leads to an indeterminacy of the form 0/0. Therefore, the derivative of each function and we get

In the numerator, the derivative of the polynomial was calculated, and in the denominator, the derivative of the complex logarithmic function. Before the last equal sign, the usual limit was calculated, substituting a deuce instead of an x.

Example 2 Calculate the limit of the ratio of two functions using L'Hospital's rule:

Example 3 Calculate the limit of the ratio of two functions using L'Hospital's rule:

Decision. Substitution in given function values x=0 leads to an indeterminacy of the form 0/0. Therefore, we calculate the derivatives of the functions in the numerator and denominator and get:

Example 4 Calculate

Decision. Substituting the value of x equal to plus infinity into a given function leads to an indeterminacy of the form ∞/∞. Therefore, we apply L'Hopital's rule:

Comment. Let's move on to examples in which the L'Hopital rule has to be applied twice, that is, to come to the limit of the ratio of the second derivatives, since the limit of the ratio of the first derivatives is an uncertainty of the form 0/0 or ∞/∞.

Example 5 Calculate the limit of the ratio of two functions using L'Hospital's rule:

Here L'Hospital's rule is applied twice, since both the limit of the ratio of functions and the limit of the ratio of derivatives give an uncertainty of the form ∞/∞.

Example 6 Calculate

Here L'Hospital's rule is applied twice, since both the limit of the ratio of functions and the limit of the ratio of derivatives give an uncertainty of the form 0/0.

Example 7 Calculate

Here L'Hopital's rule is applied twice, since both the limit of the ratio of functions and the limit of the ratio of derivatives first give an uncertainty of the form - ∞/∞, and then an uncertainty of the form 0/0.

Example 8 Calculate

Here L'Hospital's rule is applied twice, since both the limit of the ratio of functions and the limit of the ratio of derivatives first give an uncertainty of the form ∞/∞, and then an uncertainty of the form 0/0.

Apply L'Hopital's rule yourself and then see the solution

Example 9 Calculate

Clue. Here you have to puff a little more than usual on the transformation of expressions under the limit sign.

Example 10 Calculate

Clue. Here L'Hopital's rule will have to be applied three times.

Disclosure of uncertainties of the form "zero multiplied by infinity"

Example 11. Calculate

(here we have transformed the uncertainty of the form 0∙∞ to the form ∞/∞, since

and then applied L'Hopital's rules).

Example 12. Calculate

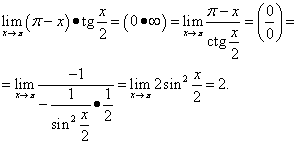
This example uses the trigonometric identity.

Disclosure of uncertainties of the types "zero to the power of zero", "infinity to the power of zero" and "one to the power of infinity"

Uncertainties of the form , or are usually reduced to the form 0/0 or ∞/∞ using the logarithm of a function of the form

To calculate the limit of an expression, one should use logarithmic identity, a special case of which is also the property of the logarithm .

Using the logarithmic identity and the continuity property of the function (to go beyond the sign of the limit), the limit should be calculated as follows:

Separately, one should find the limit of the expression in the exponent and build e to the found degree.

Example 13

Example 14 Calculate using L'Hopital's rule

Example 15 Calculate using L'Hopital's rule

Calculate the limit of the expression in the exponent

Disclosure of uncertainties of the form "infinity minus infinity"

These are cases where the calculation of the limit of the difference of functions leads to the uncertainty "infinity minus infinity": .

The calculation of such a limit according to L'Hopital's rule in general view as follows:

These transformations often result in complex expressions, therefore, it is advisable to use such transformations of the difference of functions as reduction to common denominator, multiplication and division by the same number, use trigonometric identities etc.

Example 16 Calculate using L'Hopital's rule

Example 17. Calculate using L'Hopital's rule

Calculate limits using the lopital rule

Uncertainty also does not resist turning into or:

Rules of L'Hospital

We continue to develop the topic, which was thrown to us by the member of the Paris Academy of Sciences, Marquis Guillaume Francois de Lopital. The article acquires a pronounced practical coloring and in a fairly common task it is required:

In order not to shrink, we calculate the limit of the indicator separately:

Another Papuan also gives up before the formula. In this case:

L'Hopital's rules are a very powerful method that allows you to quickly and effectively eliminate these uncertainties, it is no coincidence that in collections of problems, in tests, tests, a stable stamp is often found: "calculate the limit, without using L'Hopital's rule". Dedicated in bold requirement is possible with clear conscience assign and to any lesson limit Limits. Solution examples, Remarkable Limits. Limit Solving Methods, Remarkable Equivalences, where the uncertainty "zero to zero" or "infinity to infinity" occurs. Even if the task is formulated briefly - "calculate the limits", then it is implicitly understood that you will use anything you like, but not the rules of L'Hospital.

Metamorphoses continue, now the uncertainty “zero to zero” has come out. In principle, you can get rid of the cosine by indicating that it tends to unity. But a wise strategy is to ensure that no one gets to the bottom of anything. Therefore, we immediately apply the L'Hopital rule, as required by the condition of the problem:

A similar task for an independent solution:

As you can see, the differentiation of the numerator and denominator led us to the answer with half a turn: we found two simple derivatives, substituted “two” in them, and it turned out that the uncertainty disappeared without a trace!

Calculate the limit of a function using L'Hopital's rule

In turn, drinking companions and more exotic comrades are pulled up to the light. The transformation method is simple and standard:

The considered example is destroyed and through wonderful limits , a similar case is discussed at the end of the article Complex limits.

I’ll make a reservation right away that the rules will be given in a concise “practical” form, and if you have to pass the theory, I recommend that you turn to the textbook for more rigorous calculations.

6) Applicable last rule information to the second wonderful frontier

The disclosure of uncertainties is reduced to the previously discussed uncertainties. If, and at, then apply the transformation

infinity or zero by zero is the application of L'Hopital's rule: the limit of the ratio of two

In the case of the last three uncertainties, transformations must be applied

5) There is an indeterminacy of the form infinity to infinity.

infinitely small or two infinitely large functions is equal to the limit of the ratio of their derivatives,

3) Given the uncertainty, apply the previous rule

Calculation of limits according to L'Hopital's rule

An efficient way to calculate the limits of functions that have singularities of type infinity on

Decision. 1) By substitution, we establish that we have an uncertainty of the form zero by zero. To get rid of

Again we got the uncertainty of the form and re-apply L'Hospital's rule

2) As in the previous example, we have uncertainty. By L'Hopital's rule, we find

The application of L'Hopital's rule showed all the possibilities in disclosing uncertainties.

The number is chosen in such a way that equality (1) is satisfied and, therefore, . Thus, for a function on the interval

In the vicinity of the point x 0 , i.e. on (x 0 ,x), the conditions of the Cauchy theorem are satisfied for the functions f(x) and g(x). Therefore, there is a point сО(x 0 , x) such that

L'Hopital's rule

However, a situation is possible when the function will have an extremum at the point x 0 in the case when the derivative does not exist.

Let the function be n times differentiable in a neighborhood of the point x 0. Let's find a polynomial of degree not higher than n-1, such that

Let the functions f(x) and g(x) be continuous and differentiable in some neighborhood of the point x 0 , except for the point x 0 itself, moreover. Let be, . Then if there is a limit of the ratio of derivatives of functions, then there is a limit of the ratio of the functions themselves, and they are equal to each other, i.e. .

Conclusion: exponential function(y=a n) always grows faster than the power law (y=x n).

As an example of the application of the Maclaurin formula, we determine the number of terms in the expansion of a function in terms of formula to calculate its value with an accuracy of 0.001 for any x from the interval [-1,1].

Definition: The function is called non-decreasing (non-increasing) to (a;b) if for any x 1 Posted in Useful articles

Finding the limit of a function at a point according to L'Hopital's rule

Finding the limit of a function, according to L'Hopital's rule, revealing uncertainties of the form 0/0 and ∞/∞.

The calculator below finds the limit of the function according to the L'Hospital rule (through the derivatives of the numerator and denominator). See rule description below.

Limit of a function at a point - L'Hopital's rule

Valid Operations: + - / * ^ Constants: pi Functions: sin cosec cos tg ctg sech sec arcsin arccosec arccos arctg arcctg arcsec exp lb lg ln versin vercos haversin exsec excsc sqrt sh ch th cth csch

L'Hopital's rule

If the following conditions are met:

the limits of the functions f(x) and g(x) are equal to each other and equal to zero or infinity:
or;
the functions g(x) and f(x) are differentiable in the punctured neighborhood a;
the derivative of the function g(x) is not equal to zero in the punctured neighborhood a
and there is a limit on the ratio of the derivative f(x) to the derivative g(x):

Then there is a limit of the ratio of the functions f(x) and g(x):
,

And it is equal to the limit of the ratio of the derivative of the function f(x) to the derivative of the function g(x):

The formula allows the use of the number pi (pi), the exponent (e), the following mathematical operators:

+ - addition
— - subtraction
* - multiplication
/ - division
^ - exponentiation

and the following features:

sqrt - square root

root p- degree root p, for example root3(x) is the cube root

exp - e to the specified power

lb - base 2 logarithm

lg - base 10 logarithm

ln- natural logarithm(based on e)

log p- base logarithm p, for example log7(x) - base 7 logarithm

sin - sine

cos - cosine

tg - tangent

ctg - cotangent

sec - secant

cosec - cosecant

arcsin - arcsine

arccos - arc cosine

arctg - arc tangent

arcctg - arc tangent

arcsec - arcsecant

arccosec - arccosecant

versin - versinus

vercos - coversine

haversin - haversinus

exsec - exsecant

excsc - excosecant

sh - hyperbolic sine

ch - hyperbolic cosine

th - hyperbolic tangent

cth - hyperbolic cotangent

sech - hyperbolic secant

csch - hyperbolic cosecant

abs- absolute value(module)

sgn - signum (sign)

Rent a Gazelle or Sable Van without a driver Gazelle-Business, 1 driver + 2 passengers. Body: 3 m. length, 2 m. height, butka. The volume of the cube 10.5. Engine: UMZ-4216 (gasoline), euro-4, 106.8 […]
Details for paying taxes and contributions in 2017-2018 Details for paying taxes in 2017-2018 are an integral part of any payment. Correctly fill out the payment order […]
The procedure for consideration by the Federation Council of the adopted State Duma federal law(Articles 103–110) Article 103. Adoption of a federal law for consideration by the […]
Criminal law. General part Criminal law rule A criminal law rule is a rule of conduct established by the state that provides participants public relations […]
The size of the penalty for delay on a mortgage is limited On July 24, a law will come into force that limits the amount of the penalty for non-fulfillment or improper fulfillment of […]
Murder with aggravated circumstances punishment In accordance with the current criminal law, simple murder (part 1 of article 105 of the Criminal Code of the Russian Federation) “is punishable by imprisonment for a […]

Disclosure of uncertainties of the form 0/0 or ∞/∞ and some other uncertainties that arise in the calculation limit the relationship of two infinitesimal or infinitely large functions is greatly simplified with the help of L'Hospital's rule (actually two rules and remarks on them).

essence rules of L'Hospital is that in the case when the calculation of the limit of the ratios of two infinitely small or infinitely large functions gives uncertainties of the form 0/0 or ∞/∞, the limit of the ratio of two functions can be replaced by the limit of the ratio of their derivatives and thus get a certain result.

Let's move on to the formulation of L'Hopital's rules.

L'Hopital's Rule for the Case of the Limit of Two Infinitely Small Values. If functions f(x) and g(x aa, and in this neighborhood g"(x a equal to each other and equal to zero

().

L'Hôpital's rule for the case of the limit of two infinitely large quantities. If functions f(x) and g(x) are differentiable in some neighborhood of the point a, with the possible exception of the point a, and in this neighborhood g"(x)≠0 and if and if the limits of these functions as x tends to the value of the function at the point a equal to each other and equal to infinity

(),

then the limit of the ratio of these functions is equal to the limit of the ratio of their derivatives

().

Remarks.

1. L'Hopital's rules are also applicable when the functions f(x) and g(x) are not defined at x = a.

3. L'Hopital's rules are also applicable when the argument of the functions (x) tends to a non-finite number a, and to infinity ( x → ∞).

Uncertainties of other types can also be reduced to uncertainties of the types 0/0 and ∞/∞.

Disclosure of uncertainties of the types "zero divided by zero" and "infinity divided by infinity"

Example 1

x=2 leads to an indeterminacy of the form 0/0. Therefore, the derivative of each function and we get

In the numerator, the derivative of the polynomial was calculated, and in the denominator - derivative of a complex logarithmic function. Before the last equal sign, the usual limit, substituting a deuce instead of x.

Example 2 Calculate the limit of the ratio of two functions using L'Hospital's rule:

Decision. Substitution into a given value function x

Example 3 Calculate the limit of the ratio of two functions using L'Hospital's rule:

Decision. Substitution into a given value function x=0 leads to an indeterminacy of the form 0/0. Therefore, we calculate the derivatives of the functions in the numerator and denominator and get:

Example 4 Calculate

Decision. Substituting the value of x equal to plus infinity into a given function leads to an indeterminacy of the form ∞/∞. Therefore, we apply L'Hopital's rule:

Apply L'Hopital's rule yourself and then see the solution

Disclosure of uncertainties of the form "zero multiplied by infinity"

Example 12. Calculate

Decision. We get

This example uses the trigonometric identity.

Disclosure of uncertainties of the types "zero to the power of zero", "infinity to the power of zero" and "one to the power of infinity"

Uncertainties of the form , or are usually reduced to the form 0/0 or ∞/∞ using the logarithm of a function of the form

To calculate the limit of the expression, one should use the logarithmic identity, a special case of which is the property of the logarithm .

Using the logarithmic identity and the continuity property of the function (to go beyond the sign of the limit), the limit should be calculated as follows:

Separately, one should find the limit of the expression in the exponent and build e to the found degree.

Example 13

Decision. We get

Example 14 Calculate using L'Hopital's rule

Decision. We get

Calculate the limit of the expression in the exponent

Example 15 Calculate using L'Hopital's rule

This mathematical calculator online will help you if needed calculate function limit. Program limit solutions not only gives the answer to the problem, it leads detailed solution with explanations, i.e. displays the progress of the limit calculation.

This program can be useful for high school students general education schools in preparation for control work and exams, when testing knowledge before the exam, parents to control the solution of many problems in mathematics and algebra. Or maybe it's too expensive for you to hire a tutor or buy new textbooks? Or do you just want to get it done as soon as possible? homework math or algebra? In this case, you can also use our programs with a detailed solution.

Thus, you can carry out your own training and/or training their younger brothers or sisters, while the level of education in the field of tasks being solved increases.

Enter a function expression
Calculate Limit

It was found that some scripts needed to solve this task were not loaded, and the program may not work.
You may have AdBlock enabled.
In this case, disable it and refresh the page.

You have JavaScript disabled in your browser.
JavaScript must be enabled for the solution to appear.
Here are instructions on how to enable JavaScript in your browser.

Because There are a lot of people who want to solve the problem, your request is queued.
After a few seconds, the solution will appear below.
Wait, please sec...

If you noticed an error in the solution, then you can write about it in the Feedback Form .
Do not forget indicate which task you decide what enter in the fields.

Our games, puzzles, emulators:

A bit of theory.

The limit of the function at x-> x 0

Let the function f(x) be defined on some set X and let the point $x_0 \in X $ or $x_0 \notin X $

Take from X a sequence of points other than x 0:
x 1 , x 2 , x 3 , ..., x n , ... (1)
converging to x*. The function values at the points of this sequence also form a numerical sequence
f(x 1), f(x 2), f(x 3), ..., f(x n), ... (2)
and one can pose the question of the existence of its limit.

Definition. The number A is called the limit of the function f (x) at the point x \u003d x 0 (or at x -> x 0), if for any sequence (1) of values \u200b\u200bof the argument x that converges to x 0, different from x 0, the corresponding sequence (2) of values function converges to the number A.

$$ \lim_(x\to x_0)( f(x)) = A $$

The function f(x) can have only one limit at the point x 0. This follows from the fact that the sequence
(f(x n)) has only one limit.

There is another definition of the limit of a function.

Definition The number A is called the limit of the function f(x) at the point x = x 0 if for any number $\varepsilon > 0 $ there exists a number $\delta > 0 $ such that for all $x \in X, \; x \neq x_0 $ satisfying the inequality $|x-x_0| Using logical symbols, this definition can be written as
\((\forall \varepsilon > 0) (\exists \delta > 0) (\forall x \in X, \; x \neq x_0, \; |x-x_0| Note that the inequalities \(x \neq x_0 , \; |x-x_0| The first definition is based on the notion of a limit number sequence, which is why it is often referred to as the "sequence language" definition. The second definition is called the "language \(\varepsilon - \delta $" definition.
These two definitions of the limit of a function are equivalent, and you can use any of them, depending on which one is more convenient for solving a particular problem.

Note that the definition of the limit of a function "in the language of sequences" is also called the definition of the limit of a function according to Heine, and the definition of the limit of a function "in the language $\varepsilon - \delta $" is also called the definition of the limit of a function according to Cauchy.

Function limit at x->x 0 - and at x->x 0 +

In what follows, we will use the concepts of one-sided limits of a function, which are defined as follows.

Definition The number A is called the right (left) limit of the function f (x) at the point x 0 if for any sequence (1) converging to x 0, whose elements x n are greater (less) than x 0 , the corresponding sequence (2) converges to A.

Symbolically it is written like this:
$$ \lim_(x \to x_0+) f(x) = A \; \left(\lim_(x \to x_0-) f(x) = A \right) $$

One can give an equivalent definition of one-sided limits of a function "in the language $\varepsilon - \delta $":

Definition the number A is called the right (left) limit of the function f(x) at the point x 0 if for any $\varepsilon > 0 $ there exists $\delta > 0 $ such that for all x satisfying the inequalities \(x_0 Symbolic entries:

\((\forall \varepsilon > 0) (\exists \delta > 0) (\forall x, \; x_0

We have already begun to deal with the limits and their solution. Let's continue in hot pursuit and deal with the solution of limits according to L'Hopital's rule. This simple rule able to help you get out of the insidious and difficult traps that teachers so love to use in the examples on the control software higher mathematics and math analysis. The solution by L'Hopital's rule is simple and fast. The main thing is to be able to differentiate.

L'Hopital's Rule: History and Definition

In fact, this is not exactly L'Hopital's rule, but the rule L'Hospital-Bernoulli. Formulated by a Swiss mathematician Johann Bernoulli, and the French Guillaume Lopital first published in his textbook infinitesimals in the glorious 1696 year. Can you imagine how people had to solve the limits with the disclosure of uncertainties before this happened? We are not.

Before proceeding with the analysis of the L'Hopital rule, we recommend reading the introductory article about and methods for solving them. Often in tasks there is a wording: find the limit without using the L'Hopital rule. You can also read about the techniques that will help you with this in our article.

If you are dealing with the limits of a fraction of two functions, be prepared: you will soon meet with an uncertainty of the form 0/0 or infinity/infinity. What does it mean? In the numerator and denominator, the expressions tend to zero or infinity. What to do with such a limit, at first glance, is completely incomprehensible. However, if you apply L'Hopital's rule and think a little, everything falls into place.

But let's formulate the L'Hospital-Bernoulli rule. To be perfectly precise, it is expressed by a theorem. L'Hopital's rule, definition:

If two functions are differentiable in a neighborhood of a point x=a vanish at this point, and there is a limit to the ratio of the derivatives of these functions, then for X aspiring to a there is a limit on the ratio of the functions themselves, which is equal to the limit on the ratio of the derivatives.

Let's write down the formula, and everything will immediately become easier. L'Hopital's rule, formula:

Since we are interested in the practical side of the issue, we will not present here the proof of this theorem. You will either have to take our word for it, or find it in any calculus textbook and make sure that the theorem is correct.

By the way! For our readers there is now a 10% discount on

Disclosure of uncertainties according to L'Hopital's rule

What uncertainties can L'Hospital's rule help to uncover? Earlier we talked mainly about uncertainty 0/0 . However, this is far from the only uncertainty that can be encountered. Here are other types of uncertainties:

Let's consider the transformations that can be used to bring these uncertainties to the form 0/0 or infinity/infinity. After the transformation, it will be possible to apply the L'Hospital-Bernoulli rule and click examples like nuts.

Species Uncertainty infinity/infinity reduces to an indeterminacy of the form 0/0 simple transformation:

Let there be a product of two functions, one of which the first tends to zero, and the second - to infinity. We apply the transformation, and the product of zero and infinity turns into indeterminacy 0/0 :

To find limits with uncertainties of type infinity minus infinity we use the following transformation leading to uncertainty 0/0 :

In order to use L'Hopital's rule, you need to be able to take derivatives. Below is a table of derivatives elementary functions, which you can use when solving examples, as well as the rules for calculating derivatives of complex functions:

Now let's move on to examples.

Example 1

Find the limit by L'Hospital's rule:

Example 2

Calculate using L'Hopital's rule:

Important point! If the limit of the second and subsequent derivatives of functions exists for X aspiring to a , then L'Hopital's rule can be applied several times.

Let's find the limit ( n – natural number). To do this, apply L'Hospital's rule n once:

We wish you good luck in learning mathematical analysis. And if you need to find the limit using the L'Hopital rule, write an abstract according to the L'Hopital rule, calculate the roots differential equation or even calculate the inertia tensor of a body, please contact our authors. They will be happy to help you figure out the intricacies of the solution.